出典: フリー百科事典『ウィキペディア(Wikipedia)』
複素平面内のクラインの j -不変量
数 学 で は 複 素 変 数 τ の 函 数 で あ る フ ェ リ ッ ク ス ・ ク ラ イ ン の j - 不 変 量 ( j - i n v a r i a n t ) ︵ も し く は j - 函 数 ︶ と は 、 複 素 数 の 上 半 平 面 上 に 定 義 さ れ た S L ( 2 , Z ) の ウ ェ イ ト 0 の モ ジ ュ ラ ー 函 数 で あ る 。 j - 不 変 量 と し て 、 尖 点 で 一 位 の 極 を 持 つ 以 外 は 正 則 な 関 数 で あ り 、 次 を 満 た す も の が 一 意 に 定 ま る 。
j
(
e
2 3
π
i
)
=
0
,
j (
i )
=
1728
{\displaystyle j\left(e^{{\frac {2}{3}}\pi i}\right)=0,\quad j(i )=1728}
j の 有 理 函 数 は モ ジ ュ ラ ー で あ り 、 実 際 に す べ て の モ ジ ュ ラ ー 函 数 を 与 え る 。 古 典 的 に は 、 j - 不 変 量 は C 上 の 楕 円 曲 線 の パ ラ メ ー タ 化 と し て 研 究 さ れ て い た が 、 驚 く べ き こ と に 、 モ ン ス タ ー 群 の 対 称 性 と の 関 係 を 持 っ て い る ︵ こ の 関 係 は モ ン ス ト ラ ス ・ ム ー ン シ ャ イ ン と 呼 ば れ る ︶ 。
j - 不 変 量 は あ る 無 限 和 ︵ 下 記 の g 2 , g 3 を 参 照 ︶ で 純 粋 に 定 義 す る こ と が で き る が 、 こ れ ら は 楕 円 曲 線 の 同 型 類 を 考 え る こ と が 動 機 と な る 。 C 上 の す べ て の 楕 円 曲 線 E は 複 素 ト ー ラ ス で あ る の で 、 ラ ン ク 2 の 格 子 、 つ ま り C の 2 次 元 格 子 と 同 一 視 で き る 。 格 子 の 互 い に 平 行 な 反 対 側 の 辺 を 同 一 視 す る こ と で 、 そ の よ う に み な す こ と が で き る 。 複 素 数 を 格 子 に 掛 け る こ と は 格 子 の 回 転 や ス ケ ー リ ン グ に 対 応 し 、 こ れ ら は 楕 円 曲 線 の 同 型 類 を 保 存 す る こ と が わ か り 、 こ の こ と か ら 、 格 子 を 1 と 上 半 平 面 H の あ る 元 τ に よ っ て 生 成 さ れ る と 考 え て よ い 。 逆 に 、
g
2
=
60
∑
(
m ,
n )
≠
(
0
,
0
)
(
m +
n τ
)
−
4
,
{\displaystyle g_{2}=60\sum _{(m,n)\neq (0,0)}(m+n\tau )^{-4},}
g
3
=
140
∑
(
m ,
n )
≠
(
0
,
0
)
(
m +
n τ
)
−
6
,
{\displaystyle g_{3}=140\sum _{(m,n)\neq (0,0)}(m+n\tau )^{-6},}
と 定 義 す る と 、 こ の 格 子 は ヴ ァ イ エ ル シ ュ ト ラ ス の 楕 円 函 数 を 通 し て 、 y 2 = 4 x 3 − g 2 x - g 3 で 定 義 さ れ た C 上 の 楕 円 曲 線 に 対 応 す る 。 こ の と き 、 j - 不 変 量 は 、
j (
τ
)
=
1728
g
2
3
Δ
{\displaystyle j(\tau )=1728\,{\frac {g_{2}^{3}}{\Delta }}}
と 定 義 さ れ る 。 こ こ に モ ジ ュ ラ ー 判 別 式 ( m o d u l a r d i s c r i m i n a n t ) Δ は
Δ
=
g
2
3
−
27
g
3
2
{\displaystyle \Delta =g_{2}^{3}-27g_{3}^{2}}
で あ る 。
Δ は ウ ェ イ ト 12 の モ ジ ュ ラ ー 形 式 で あ る こ と と 、 g 2 は ウ ェ イ ト 4 の モ ジ ュ ラ ー 形 式 で あ る の で そ の 3 乗 は ウ ェ イ ト 12 で あ る こ と を 示 す こ と が で き る 。 し た が っ て 、 j が こ れ ら の 商 で あ る こ と か ら j は ウ ェ イ ト 0 の モ ジ ュ ラ 函 数 で あ り 、 特 に 、 S L ( 2 , Z ) の 作 用 の 下 に 不 変 な 有 理 型 函 数 H → C で あ る 。 以 下 に 説 明 す る よ う に j は 全 射 で あ り 、 こ の こ と は C 上 の 楕 円 曲 線 の 同 型 類 と 複 素 数 の 間 の 全 単 射 を 与 え る こ と を 意 味 す る 。
基 本 領 域 [ 編 集 ] 上 半 平 面 上 に 作 用 す る モ ジ ュ ラ 群 の 基 本 領 域 2 つ の 変 換 τ → τ + 1 と τ → - τ − 1 は モ ジ ュ ラ 群 と 呼 ば れ る 群 を 生 成 し 、 こ の 群 は 射 影 特 殊 線 型 群 P S L ( 2 , Z ) と 同 一 視 で き る 。 こ の 群 に 属 す る 適 当 な 変 換
τ
↦
a τ
+
b
c τ
+
d
,
a d −
b c =
1 ,
{\displaystyle \tau \mapsto {\frac {a\tau +b}{c\tau +d}},\qquad ad-bc=1,}
を 選 択 す る こ と に よ り 、 τ を j の 基 本 領 域 ︵ 英 語 版 ︶ ( f u n d a m e n t a l r e g i o n ) 内 に あ り j に 対 し て 同 じ 値 を と る 、 あ る 値 に 帰 着 さ せ る こ と が で き る 。 基 本 領 域 は 次 の 条 件 を 満 た す τ か ら 構 成 さ れ て い る 。
|
τ
|
≥
1
−
1 2
<
R
(
τ
)
≤
1 2
−
1 2
<
R
(
τ
)
<
0
⇒
|
τ
|
>
1
.
{\displaystyle {\begin{aligned}|\tau |&\geq 1\\-{\tfrac {1}{2}}&<{\mathfrak {R}}(\tau )\leq {\tfrac {1}{2}}\\-{\tfrac {1}{2}}&<{\mathfrak {R}}(\tau )<0\Rightarrow |\tau |>1\end{aligned}}.}
函 数 j ( τ ) を こ の 領 域 へ 制 限 す る と 、 複 素 数 C の す べ て の 値 を ち ょ う ど 一 度 だ け 取 る 。 言 い 換 え る と 、 C す べ て の 元 c に 対 し 、 c = j ( τ ) と な る 基 本 領 域 の 元 τ が 一 意 に 存 在 す る 。 こ の よ う に 、 j は 基 本 領 域 を 全 複 素 平 面 へ 写 像 す る と い う 性 質 を 持 っ て い る 。
リ ー マ ン 面 と し て 、 基 本 領 域 の 種 数 は 0 で あ り 、 す べ て の ︵ レ ベ ル 1 の ︶ モ ジ ュ ラ ー 函 数 は j の 有 理 函 数 で あ り 、 逆 に 、 j の す べ て の 有 理 函 数 は モ ジ ュ ラ ー 函 数 で あ る 。 言 い 換 え る と 、 モ ジ ュ ラ ー 函 数 全 体 の な す 体 は C ( j ) で あ る 。
類 体 論 と j - 不 変 量 [ 編 集 ] j - 不 変 量 は 、 多 く の 注 目 す べ き 性 質 を 有 す る 。
● τ が 虚 数 乗 法 で あ る 、 す な わ ち 、 虚 数 部 が 正 で あ る 虚 二 次 体 の 任 意 の 元 で あ る ︵ 従 っ て 、 j - 不 変 量 が 定 義 さ れ る ︶ な ら ば 、 j ( τ ) は 代 数 的 整 数 で あ る [ 1 ] 。 ● 体 の 拡 大 Q [ j ( τ ) , τ ] / Q ( τ ) は ア ー ベ ル 的 、 す な わ ち 、 ガ ロ ア 群 が ア ー ベ ル 的 に な る 。 ● Λ を { 1 , τ } で 生 成 さ れ る C の 中 の 格 子 と す る と 、 乗 法 の 下 に Λ を 固 定 す る Q ( τ ) の す べ て の 元 が 、 整 環 ︵ 英 語 版 ︶ ( o r d e r ) と 呼 ば れ る 環 の 単 位 元 ( u n i t ) を 形 成 す る こ と が 容 易 に わ か る 。 同 様 に 、 同 じ 整 環 の 生 成 子 { 1 , τ ′ } を 持 つ 格 子 は 、 Q ( τ ) 上 で j ( τ ) の 代 数 的 共 役 で あ る j ( τ ' ) を 定 義 す る 。 包 含 関 係 に 従 い 、 Q ( τ ) の 唯 一 の 最 大 整 環 は 、 Q ( τ ) の 代 数 的 整 数 の 環 で あ り 、 そ の 環 を 持 つ τ の 値 は 、 Q ( τ ) の 不 分 岐 拡 大 を 導 く 。 こ れ ら の 古 典 的 な 結 果 は 、 虚 数 乗 法 論 の 出 発 点 と な っ て い る 。
超 越 的 性 質 [ 編 集 ] 1 9 3 7 年 、 テ オ ド ー ル ・ シ ュ ナ イ ダ ー ︵ 英 語 版 ︶ ( T h e o d o r S c h n e i d e r ) は 、 前 述 の τ が 上 半 平 面 で 二 次 の 無 理 数 で あ れ ば j ( τ ) は 代 数 的 数 で あ る と い う こ と を 証 明 し た 。 加 え て 、 τ が 代 数 的 数 だ が 虚 二 次 体 の 数 で な い な ら ば 、 j ( τ ) は 超 越 数 で あ る こ と を も 証 明 し た 。
j - 函 数 は 数 多 く の 超 越 的 性 質 を 持 つ 。 ク ル ト ・ マ ー ラ ー ︵ 英 語 版 ︶ ( K u r t M a h l e r ) は マ ー ラ ー 予 想 と も 呼 ば れ る 特 別 な 超 越 性 を 予 想 し 、 1 9 9 0 年 代 に ユ ー リ ・ ネ ス テ レ ン コ ( Y u . V . N e s t e r n k o ) と パ ト リ ス ・ フ ィ リ ポ ン ( P a t r i c e P h i l l i p o n ) の 結 果 の 系 と し て 証 明 さ れ た 。 マ ー ラ ー 予 想 と は 、 τ が 上 半 平 面 に あ れ ば e x p ( 2 π i τ ) と j ( τ ) は 双 方 が 同 時 に 代 数 的 に は な ら な い で あ ろ う と い う 予 想 で あ る 。 現 在 は よ り 強 い 結 果 が 知 ら れ て い て 、 例 え ば 、 e x p ( 2 π i τ ) が 代 数 的 で あ れ ば 次 の 3 つ の 数 は 代 数 的 に 独 立 で 、 超 越 数 に な る 。
j (
τ
)
,
j
′
(
τ
)
π
,
j
′
′
(
τ
)
π
2
.
{\displaystyle j(\tau ),{\frac {j^{\prime }(\tau )}{\pi }},{\frac {j^{\prime \prime }(\tau )}{\pi ^{2}}}.}
q - 展 開 と ム ー ン シ ャ イ ン [ 編 集 ] j の 注 目 す べ き 性 質 の い く つ か は 、 q = e x p ( 2 π i τ ) で の ロ ー ラ ン 級 数 と し て 書 か れ る q - 展 開 ︵ フ ー リ エ 級 数 展 開 ︶ に 関 連 し て い る 。 q - 展 開 は 、
j (
τ
)
=
1 q
+
744
+
196884
q +
21493760
q
2
+
864299970
q
3
+
20245856256
q
4
+
⋯
{\displaystyle j(\tau )={1 \over q}+744+196884q+21493760q^{2}+864299970q^{3}+20245856256q^{4}+\cdots }
で 始 ま っ て い る 。
な お 、 j は 尖 点 で 一 位 の 単 純 極 を 持 つ の で 、 q - 展 開 に は q − 1 未 満 の 項 が な い 。
こ の フ ー リ エ 係 数 は す べ て 整 数 で あ り 、 こ の こ と が い く つ か の 概 整 数 、 例 え ば 有 名 な ラ マ ヌ ジ ャ ン 定 数 ︵ 英 語 版 ︶ ( R a m a n u j a n ' s c o n s t a n t ) の 理 由 と な る 。
e
π
163
≈
640320
3
+
744
{\displaystyle e^{\pi {\sqrt {163}}}\approx 640320^{3}+744}
q n の 係 数 の 漸 近 公 式 ︵ 英 語 版 ︶ ( a s y m p t o t i c f o r m u l a ) は 、 ハ ー デ ィ ・ リ ト ル ウ ッ ド の 円 周 法 ︵ 英 語 版 ︶ ( H a r d y – L i t t l e w o o d c i r c l e m e t h o d ) で 示 す こ と が で き た よ う に 、
e
4 π
n
2
n
3
/
4
{\displaystyle {\frac {e^{4\pi {\sqrt {n}}}}{{\sqrt {2}}n^{3/4}}}}
, に よ り 与 え ら れ る 。 [ 2 ] [ 3 ]
ムーンシャイン [ 編集 ]
さ ら に 注 目 す べ き は 、 q の 正 の べ き 乗 の 項 の フ ー リ エ 係 数 が ム ー ン シ ャ イ ン 加 群 ︵ 英 語 版 ︶ ( m o o n s h i n e m o d u l e ) と 呼 ば れ る モ ン ス タ ー 群 の 無 限 次 元 次 数 付 き 代 数 表 現 の 次 数 部 の 次 元 で あ る こ と で あ る 。 特 に 、 q n の 係 数 は 、 ム ー シ ャ イ ン 加 群 の 次 数 n の 次 元 と な っ て い る 。 第 一 の 例 は グ ラ イ ス 代 数 ︵ 英 語 版 ︶ ( G r i e s s a l g e b r a ) で あ り 、 こ の 代 数 は 次 元 1 9 6 , 8 8 4 で 、 項 1 9 6 8 8 4 q に 対 応 し て い る 。 こ の 驚 く べ き 観 察 が ム ー ン シ ャ イ ン 理 論 の 出 発 点 で あ っ た 。
ム ー ン シ ャ イ ン 予 想 の 研 究 は 、 ジ ョ ン ・ ホ ー ト ン ・ コ ン ウ ェ イ と シ モ ン ・ ノ ー ト ン ︵ 英 語 版 ︶ ( S i m o n P . N o r t o n ) に よ り 種 数 0 の モ ジ ュ ラ 函 数 を 見 つ け る こ と に 発 展 し た 。 ジ ョ ン ・ G ・ ト ン プ ソ ン は 、
q
−
1
+
O
(
q )
{\displaystyle q^{-1}+{O}(q )}
と い う 形 式 に 正 規 化 さ れ る 種 数 0 の モ ジ ュ ラ 函 数 が 、 有 限 個 し か 存 在 し な い こ と を 証 明 し た 。
別 の 表 現 [ 編 集 ] λ を モ ジ ュ ラ ラ ム ダ 函 数 ︵ 英 語 版 ︶ ( m o d u l a r l a m b d a f u n c t i o n ) と し 、 x = λ ( 1 − λ ) と 置 く と
j (
τ
)
=
256
(
1 −
x
)
3
x
2
{\displaystyle j(\tau )={\frac {256(1-x)^{3}}{x^{2}}}}
を 得 る 。
λ
(
τ
)
=
θ
2
4
(
0
,
τ
)
θ
3
4
(
0
,
τ
)
=
k
2
(
τ
)
{\displaystyle \lambda (\tau )={\frac {\theta _{2}^{4}(0,\tau )}{\theta _{3}^{4}(0,\tau )}}=k^{2}(\tau )}
は 、 ヤ コ ビ の テ ー タ 函 数
θ
m
{\displaystyle \theta _{m}}
の 比 率 で あ り 、 楕 円 モ ジ ュ ラ ス
k (
τ
)
{\displaystyle k(\tau )}
の 二 乗 で あ る 。 [ 4 ] λ が 次 の 非 調 和 比 ( c r o s s - r a t i o ) の 6 つ の 値 で 入 れ 替 わ る と き は 、 j の 値 は 不 変 で あ る [ 5 ] 。
{
λ
,
1
1 −
λ
,
λ
−
1
λ
,
1 λ
,
λ
λ
−
1
,
1 −
λ
}
.
{\displaystyle \left\lbrace {\lambda ,{\frac {1}{1-\lambda }},{\frac {\lambda -1}{\lambda }},{\frac {1}{\lambda }},{\frac {\lambda }{\lambda -1}},1-\lambda }\right\rbrace .}
j の 分 岐 点 は { 0 , 1 , ∞ } で あ る の で 、 ベ リ イ 函 数 ︵ 英 語 版 ︶ ( B e l y i f u n c t i o n ) で あ る [ 6 ] 。
テ ー タ 函 数 に よ る 表 現 [ 編 集 ]
q =
e
π
i τ
{\displaystyle q=e^{\pi i\tau }}
︵ ノ ー ム ︶ と 定 義 し 直 す と 、 ヤ コ ビ の テ ー タ 函 数
ϑ
(
0
;
τ
)
=
ϑ
00
(
0
;
τ
)
=
1 +
2
∑
n =
1
∞
(
e
π
i τ
)
n
2
=
∑
n =
−
∞
∞
q
n
2
{\displaystyle \vartheta (0;\tau )=\vartheta _{00}(0;\tau )=1+2\sum _{n=1}^{\infty }\left(e^{\pi i\tau }\right)^{n^{2}}=\sum _{n=-\infty }^{\infty }q^{n^{2}}}
か ら 指 標 付 き テ ー タ 函 数 を 導 く こ と が で き る 。 次 の よ う に 置 く こ と と す る 。
a =
θ
2
(
0
;
q )
=
ϑ
10
(
0
;
τ
)
{\displaystyle a=\theta _{2}(0;q)=\vartheta _{10}(0;\tau )}
b =
θ
3
(
0
;
q )
=
ϑ
00
(
0
;
τ
)
{\displaystyle b=\theta _{3}(0;q)=\vartheta _{00}(0;\tau )}
c =
θ
4
(
0
;
q )
=
ϑ
01
(
0
;
τ
)
{\displaystyle c=\theta _{4}(0;q)=\vartheta _{01}(0;\tau )}
こ こ に
θ
m
{\displaystyle \theta _{m}}
と
ϑ
n
{\displaystyle \vartheta _{n}}
は 記 法 を 変 え た も の と し た 。 す る と 、 ヴ ァ イ エ ル シ ュ ト ラ ス 定 数 g 2 , g 3 と デ デ キ ン ト の エ ー タ 函 数 η ( τ ) に 対 し て 、
g
2
(
τ
)
=
2 3
π
4
(
a
8
+
b
8
+
c
8
)
{\displaystyle g_{2}(\tau )={\tfrac {2}{3}}\pi ^{4}\left(a^{8}+b^{8}+c^{8}\right)}
g
3
(
τ
)
=
4 27
π
6
(
a
8
+
b
8
+
c
8
)
3
−
54 (
a b c
)
8
2
{\displaystyle g_{3}(\tau )={\tfrac {4}{27}}\pi ^{6}{\sqrt {\frac {(a^{8}+b^{8}+c^{8})^{3}-54(abc)^{8}}{2}}}}
Δ
=
g
2
3
−
27
g
3
2
=
(
2 π
)
12
η
(
τ
)
24
=
(
2 π
)
12
(
1 2
a b c
)
8
{\displaystyle \Delta =g_{2}^{3}-27g_{3}^{2}=(2\pi )^{12}\eta (\tau )^{24}=(2\pi )^{12}\left({\tfrac {1}{2}}abc\right)^{8}}
と な る 。 こ の よ う に す る と 、 j ( τ ) を 早 く 計 算 で き る 形 に 書 き 換 え る こ と が で き る 。
j (
τ
)
=
1728
g
2
3
g
2
3
−
27
g
3
2
=
32
(
a
8
+
b
8
+
c
8
)
3
(
a b c
)
8
.
{\displaystyle j(\tau )=1728{\frac {g_{2}^{3}}{g_{2}^{3}-27g_{3}^{2}}}=32{(a^{8}+b^{8}+c^{8})^{3} \over (abc)^{8}}.}
た だ し 、
a
4
−
b
4
+
c
4
=
0
{\displaystyle a^{4}-b^{4}+c^{4}=0}
で あ る こ と に 注 意 す る 。
代 数 的 定 義 [ 編 集 ] 今 ま で は 、 j を 複 素 変 数 の 函 数 と し て 考 え て き た が 、 楕 円 曲 線 の 同 型 類 の 不 変 量 と し て は 、 j を 純 粋 に 代 数 的 に 定 義 す る こ と も で き る 。
y
2
+
a
1
x y +
a
3
y =
x
3
+
a
2
x
2
+
a
4
x +
a
6
{\displaystyle y^{2}+a_{1}xy+a_{3}y=x^{3}+a_{2}x^{2}+a_{4}x+a_{6}}
を 任 意 の 体 の 上 の 平 面 楕 円 曲 線 と す る と 、
b
2
=
a
1
2
+
4
a
2
,
b
4
=
a
1
a
3
+
2
a
4
{\displaystyle b_{2}=a_{1}^{2}+4a_{2},\quad b_{4}=a_{1}a_{3}+2a_{4}}
b
6
=
a
3
2
+
4
a
6
,
b
8
=
a
1
2
a
6
−
a
1
a
3
a
4
+
a
2
a
3
2
+
4
a
2
a
6
−
a
4
2
{\displaystyle b_{6}=a_{3}^{2}+4a_{6},\quad b_{8}=a_{1}^{2}a_{6}-a_{1}a_{3}a_{4}+a_{2}a_{3}^{2}+4a_{2}a_{6}-a_{4}^{2}}
c
4
=
b
2
2
−
24
b
4
,
c
6
=
−
b
2
3
+
36
b
2
b
4
−
216
b
6
{\displaystyle c_{4}=b_{2}^{2}-24b_{4},\quad c_{6}=-b_{2}^{3}+36b_{2}b_{4}-216b_{6}}
と 定 義 す る こ と が で き 、
Δ
=
−
b
2
2
b
8
+
9
b
2
b
4
b
6
−
8
b
4
3
−
27
b
6
2
{\displaystyle \Delta =-b_{2}^{2}b_{8}+9b_{2}b_{4}b_{6}-8b_{4}^{3}-27b_{6}^{2}}
と 表 す と 、 こ れ は 楕 円 曲 線 の 判 別 式 を 表 し て い る 。
こ こ で 、 楕 円 曲 線 の j - 不 変 量 を
j =
c
4
3
Δ
{\displaystyle j={c_{4}^{3} \over \Delta }}
と 定 義 す る 。
楕 円 曲 線 が 定 義 さ れ て い る 体 の 標 数 が 2 も し く は 3 で な い 場 合 に 、 こ の 定 義 は
j =
1728
c
4
3
c
4
3
−
c
6
2
{\displaystyle j=1728{c_{4}^{3} \over c_{4}^{3}-c_{6}^{2}}}
と 書 き 直 す こ と が で き る 。
逆 函 数 [ 編 集 ] j - 不 変 量 の 逆 函 数 は 、 超 幾 何 函 数 2 F 1 で 表 す こ と も で き る ︵ ピ カ ー ル ・ フ ッ ク ス 方 程 式 ︵ 英 語 版 ︶ ( P i c a r d – F u c h s e q u a t i o n ) も 参 照 ︶ 。 与 え ら れ た 数 値 N に 対 し て 式 j ( τ ) = N を τ に つ い て 解 く た め に は 、 少 な く と も 4 つ の 方 法 が 知 ら れ て い る 。
方 法 1 : モ ジ ュ ラ ラ ム ダ 函 数 ︵ 英 語 版 ︶ ( m o d u l a r l a m b d a f u n c t i o n ) λ の 6 次 式 を 解 く 方 法 。
j (
τ
)
=
256
(
1 −
λ
(
1 −
λ
)
)
3
(
λ
(
1 −
λ
)
)
2
.
{\displaystyle j(\tau )={\frac {256(1-\lambda (1-\lambda ))^{3}}{(\lambda (1-\lambda ))^{2}}}.}
x = λ ( 1 − λ ) と す る と 6 次 式 は x の 3 次 式 と な る 。 す る と 、 λ の 6 つ の 値 の ど れ に 対 し て も 、
τ
=
i
2
F
1
(
1 2
,
1 2
,
1 ,
1 −
λ
)
2
F
1
(
1 2
,
1 2
,
1 ,
λ
)
{\displaystyle \tau =i\ {\frac {{}_{2}F_{1}\left({\tfrac {1}{2}},{\tfrac {1}{2}},1,1-\lambda \right)}{{}_{2}F_{1}\left({\tfrac {1}{2}},{\tfrac {1}{2}},1,\lambda \right)}}}
と な る 。
方 法 2 : γ の 4 次 式 を 解 く 方 法 。
j (
τ
)
=
27 (
1 +
8 γ
)
3
γ
(
1 −
γ
)
3
.
{\displaystyle j(\tau )={\frac {27(1+8\gamma )^{3}}{\gamma (1-\gamma )^{3}}}.}
任 意 の 4 つ の 根 に 対 し て 、
τ
=
i
3
2
F
1
(
1 3
,
2 3
,
1 ,
1 −
γ
)
2
F
1
(
1 3
,
2 3
,
1 ,
γ
)
{\displaystyle \tau ={\frac {i}{\sqrt {3}}}{\frac {{}_{2}F_{1}\left({\tfrac {1}{3}},{\tfrac {2}{3}},1,1-\gamma \right)}{{}_{2}F_{1}\left({\tfrac {1}{3}},{\tfrac {2}{3}},1,\gamma \right)}}}
と な る 。
方 法 3 : β の 3 次 式 を 解 く 方 法 。
j (
τ
)
=
64 (
1 +
3 β
)
3
β
(
1 −
β
)
2
.
{\displaystyle j(\tau )={\frac {64(1+3\beta )^{3}}{\beta (1-\beta )^{2}}}.}
す る と 、 任 意 の 3 つ の 根 に 対 し 、
τ
=
i
2
2
F
1
(
1 4
,
3 4
,
1 ,
1 −
β
)
2
F
1
(
1 4
,
3 4
,
1 ,
β
)
{\displaystyle \tau ={\frac {i}{\sqrt {2}}}{\frac {{}_{2}F_{1}\left({\tfrac {1}{4}},{\tfrac {3}{4}},1,1-\beta \right)}{{}_{2}F_{1}\left({\tfrac {1}{4}},{\tfrac {3}{4}},1,\beta \right)}}}
と な る 。
方 法 4 : α の 2 次 式 を 解 く 方 法 。
j (
τ
)
=
1728
4 α
(
1 −
α
)
.
{\displaystyle j(\tau )={\frac {1728}{4\alpha (1-\alpha )}}.}
す る と 、
τ
=
i
2
F
1
(
1 6
,
5 6
,
1 ,
1 −
α
)
2
F
1
(
1 6
,
5 6
,
1 ,
α
)
{\displaystyle \tau =i\ {\frac {{}_{2}F_{1}\left({\tfrac {1}{6}},{\tfrac {5}{6}},1,1-\alpha \right)}{{}_{2}F_{1}\left({\tfrac {1}{6}},{\tfrac {5}{6}},1,\alpha \right)}}}
と な る 。
2 つ の 根 は τ と - 1 / τ で あ る が 、 j ( τ ) = j ( - 1 / τ ) で あ る た め に 、 ど の α を 選 ん で も 差 異 は な い 。 後 半 3 つ の 方 法 は 、 ラ マ ヌ ジ ャ ン の 交 代 基 底 に つ い て の 楕 円 函 数 論 で 発 見 さ れ た 。
逆 函 数 は 、 こ れ ら の 根 の 比 率 が 有 界 で な い に も か か わ ら ず 、 楕 円 函 数 の 周 期 の 高 精 度 な 計 算 を 通 し て 、 う ま く 適 用 す る こ と が 可 能 で あ る 。 ま た 、 関 連 す る 帰 結 と し て 、 2 の べ き の 大 き さ を も つ 虚 数 軸 上 の 点 で j の 値 が 二 次 の 根 と な る こ と を 通 し て ︵ 逆 関 数 を ︶ 表 す こ と が で き る ︵ こ の よ う に し て 、 定 規 と コ ン パ ス に よ る 作 図 が 可 能 と な る ︶ 。 レ ベ ル が 2 の モ ジ ュ ラ 函 数 は 3 次 式 で あ る の で 、 こ の 結 果 は 自 明 で は な い 。
π 公 式 [ 編 集 ] チ ュ ダ ノ フ ス キ ー 兄 弟 ︵ 英 語 版 ︶ ( C h u d n o v s k y b r o t h e r s ) は 、 1 9 8 7 年 に 、
1 π
=
12
640320
3
/
2
∑
k =
0
∞
(
6 k )
! (
163
⋅
3344418
k +
13591409
)
(
3 k )
! (
k !
)
3
(
−
640320
)
3 k
{\displaystyle {\frac {1}{\pi }}={\frac {12}{640320^{3/2}}}\sum _{k=0}^{\infty }{\frac {(6k)!(163\cdot 3344418k+13591409)}{(3k)!(k!)^{3}(-640320)^{3k}}}}
を 発 見 し 、
j
(
1 +
−
163
2
)
=
−
640320
3
{\displaystyle j{\big (}{\tfrac {1+{\sqrt {-163}}}{2}}{\big )}=-640320^{3}}
と い う 事 実 を 示 す こ と に 使 用 し た 。 同 様 な 公 式 は 、 ラ マ ヌ ジ ャ ン ・ 佐 藤 級 数 ︵ 英 語 版 ︶ ( R a m a n u j a n - S a t o s e r i e s ) を 参 照 。
ボ ー チ ャ ー ズ の 積 公 式 [ 編 集 ] 次 は リ チ ャ ー ド ・ ボ ー チ ャ ー ズ に よ っ て 発 見 さ れ た [ 7 ] 。
j (
τ
)
−
j (
τ
′
)
=
1 q
∏
n ,
m =
1
∞
(
1 −
q
n
q ′
m
)
c
n m
{\displaystyle j(\tau )-j(\tau ')={1 \over q}\prod _{n,m=1}^{\infty }(1-q^{n}{q'}^{m})^{c_{nm}}}
で あ る ︵ こ こ で c _ n は j 関 数 の q 展 開 に お け る q ^ n の 係 数 ︶ .
特 殊 値 [ 編 集 ] j - 不 変 量 は 、 基 本 領 域 ︵ 英 語 版 ︶ ( f u n d a m e n t a l d o m a i n ) の ﹁ 角 ﹂
1 2
(
1 +
i
3
)
{\displaystyle {\tfrac {1}{2}}\left(1+i{\sqrt {3}}\right)}
で は 0 と な る 。
以 下 に 、 い く つ か の 特 殊 値 を 示 す ︵ J = j / 1 7 2 8 を 使 っ て 表 示 し て い る ︶ [ 疑 問 点 – ノ ー ト ] 。
J (
i )
=
J
(
1 +
i
2
)
=
1
J
(
2
i
)
=
(
5 3
)
3
J (
2 i )
=
(
11 2
)
3
J
(
2
2
i
)
=
125
216
(
19 +
13
2
)
3
J (
4 i )
=
1 64
(
724
+
513
2
)
3
J
(
1 +
2 i
2
)
=
1 64
(
724
−
513
2
)
3
J
(
1 +
2
2
i
3
)
=
125
216
(
19 −
13
2
)
3
J (
3 i )
=
1 27
(
2 +
3
)
2
(
21 +
20
3
)
3
J
(
2
3
i
)
=
125
16
(
30 +
17
3
)
3
J
(
1 +
7
3
i
2
)
=
−
64000
7
(
651
+
142
21
)
3
J
(
1 +
3
11
i
10
)
=
64 27
(
23 −
4
33
)
2
(
−
77 +
15
33
)
3
J
(
21
i
)
=
1 128
(
3 +
7
)
5
(
17 +
7
3
+
59
7
+
35
21
)
3
J
(
30
i
1
)
=
1 4
(
7 +
5
2
+
3
5
+
2
10
)
4
(
55 +
30
2
+
12
5
+
10
10
)
3
J
(
30
i
2
)
=
1 4
(
7 +
5
2
−
3
5
−
2
10
)
4
(
55 +
30
2
−
12
5
−
10
10
)
3
J
(
30
i
5
)
=
1 4
(
7 −
5
2
+
3
5
−
2
10
)
4
(
55 −
30
2
+
12
5
−
10
10
)
3
J
(
30
i
10
)
=
1 4
(
7 −
5
2
−
3
5
+
2
10
)
4
(
55 −
30
2
−
12
5
+
10
10
)
3
J
(
1 +
31
i
2
)
=
(
1 −
(
1 +
19
2
(
13 −
93
13 +
93
⋅
31
+
27
31
−
27
3
+
13 +
93
13 −
93
⋅
31
−
27
31
+
27
3
)
)
2
)
3
J (
5 i )
=
(
1 +
9 4
5
(
13 +
5
5
)
2
)
3
J
(
5 i +
1
2
)
=
(
1 −
9 4
5
(
13 −
5
5
)
2
)
3
J (
6 i )
=
1 216
(
2 +
3
)
10
(
231
+
380
3
+
(
204
+
158
3
)
12
4
)
3
J (
70
i )
=
(
1 +
9 4
(
303
+
220
2
+
139
5
+
96
10
)
2
)
3
J (
94
i )
=
(
1 +
9 8192
(
3 +
2
2
+
9 +
8
2
)
8
(
8 +
(
−
1 −
2
+
9 +
8
2
)
(
−
2 +
2
2
+
3 +
4
2
+
3
9 +
8
2
)
)
2
)
3
J (
7 i )
=
(
1 +
9 32
28
4
(
3 +
7
)
3
(
13 +
3
7
+
(
6 +
7
)
28
4
)
2
)
3
J (
8 i )
=
(
1 +
9 4
2
4
(
1 +
2
)
(
123
+
104
2
4
+
88
2
+
73
8
4
)
2
)
3
J (
10 i )
=
(
1 +
9 8
(
2402
+
1607
5
4
+
1074
25
4
+
719
125
4
)
2
)
3
J
(
5 i
2
)
=
(
1 +
9 8
(
2402
−
1607
5
4
+
1074
25
4
−
719
125
4
)
2
)
3
J (
130
i )
=
(
1 +
9 4
(
7392
+
3289
5
+
2040
13
+
917
65
)
2
)
3
J (
190
i )
=
(
1 +
18
(
31570
+
22323
2
+
14139
5
+
9998
10
)
2
)
3
J (
2
58
i )
=
(
1 +
9 256
(
1 +
2
)
5
(
5 +
29
)
5
(
793
+
907
2
+
237
29
+
103
58
)
2
)
3
J
(
1 +
1435
i
2
)
=
(
1 −
9
(
9892538
+
4424079
5
+
1544955
41
+
690925
205
)
2
)
3
J
(
1 +
1555
i
2
)
=
(
1 −
9
(
22297077
+
9971556
5
+
(
3571365
+
1597163
5
)
31 +
21
5
2
)
2
)
3
{\displaystyle {\begin{aligned}J(i )&=J\left({\tfrac {1+i}{2}}\right)=1\\J\left({\sqrt {2}}i\right)&={\big (}{\tfrac {5}{3}}{\big )}^{3}\\J(2i)&={\big (}{\tfrac {11}{2}}{\big )}^{3}\\J\left(2{\sqrt {2}}i\right)&={\tfrac {125}{216}}\left(19+13{\sqrt {2}}\right)^{3}\\J(4i)&={\tfrac {1}{64}}\left(724+513{\sqrt {2}}\right)^{3}\\J\left({\tfrac {1+2i}{2}}\right)&={\tfrac {1}{64}}\left(724-513{\sqrt {2}}\right)^{3}\\J\left({\tfrac {1+2{\sqrt {2}}i}{3}}\right)&={\tfrac {125}{216}}\left(19-13{\sqrt {2}}\right)^{3}\\J(3i)&={\tfrac {1}{27}}\left(2+{\sqrt {3}}\right)^{2}\left(21+20{\sqrt {3}}\right)^{3}\\J\left(2{\sqrt {3}}i\right)&={\tfrac {125}{16}}\left(30+17{\sqrt {3}}\right)^{3}\\J\left({\tfrac {1+7{\sqrt {3}}i}{2}}\right)&=-{\tfrac {64000}{7}}\left(651+142{\sqrt {21}}\right)^{3}\\J\left({\tfrac {1+3{\sqrt {11}}i}{10}}\right)&={\tfrac {64}{27}}\left(23-4{\sqrt {33}}\right)^{2}\left(-77+15{\sqrt {33}}\right)^{3}\\J\left({\sqrt {21}}i\right)&={\tfrac {1}{128}}\left(3+{\sqrt {7}}\right)^{5}\left(17+7{\sqrt {3}}+59{\sqrt {7}}+35{\sqrt {21}}\right)^{3}\\J\left({\tfrac {{\sqrt {30}}i}{1}}\right)&={\tfrac {1}{4}}\left(7+5{\sqrt {2}}+3{\sqrt {5}}+2{\sqrt {10}}\right)^{4}\left(55+30{\sqrt {2}}+12{\sqrt {5}}+10{\sqrt {10}}\right)^{3}\\J\left({\tfrac {{\sqrt {30}}i}{2}}\right)&={\tfrac {1}{4}}\left(7+5{\sqrt {2}}-3{\sqrt {5}}-2{\sqrt {10}}\right)^{4}\left(55+30{\sqrt {2}}-12{\sqrt {5}}-10{\sqrt {10}}\right)^{3}\\J\left({\tfrac {{\sqrt {30}}i}{5}}\right)&={\tfrac {1}{4}}\left(7-5{\sqrt {2}}+3{\sqrt {5}}-2{\sqrt {10}}\right)^{4}\left(55-30{\sqrt {2}}+12{\sqrt {5}}-10{\sqrt {10}}\right)^{3}\\J\left({\tfrac {{\sqrt {30}}i}{10}}\right)&={\tfrac {1}{4}}\left(7-5{\sqrt {2}}-3{\sqrt {5}}+2{\sqrt {10}}\right)^{4}\left(55-30{\sqrt {2}}-12{\sqrt {5}}+10{\sqrt {10}}\right)^{3}\\J\left({\tfrac {1+{\sqrt {31}}i}{2}}\right)&=\left(1-\left(1+{\frac {\sqrt {19}}{2}}\left({\sqrt {\tfrac {13-{\sqrt {93}}}{13+{\sqrt {93}}}}}\cdot {\sqrt[{3}]{\tfrac {{\sqrt {31}}+{\sqrt {27}}}{{\sqrt {31}}-{\sqrt {27}}}}}+{\sqrt {\tfrac {13+{\sqrt {93}}}{13-{\sqrt {93}}}}}\cdot {\sqrt[{3}]{\tfrac {{\sqrt {31}}-{\sqrt {27}}}{{\sqrt {31}}+{\sqrt {27}}}}}\right)\right)^{2}\right)^{3}\\J(5i)&=\left(1+{\tfrac {9}{4}}{\sqrt {5}}\left(13+5{\sqrt {5}}\right)^{2}\right)^{3}\\J\left({\tfrac {5i+1}{2}}\right)&=\left(1-{\tfrac {9}{4}}{\sqrt {5}}\left(13-5{\sqrt {5}}\right)^{2}\right)^{3}\\J(6i)&={\tfrac {1}{216}}\left(2+{\sqrt {3}}\right)^{10}\left(231+380{\sqrt {3}}+\left(204+158{\sqrt {3}}\right){\sqrt[{4}]{12}}\right)^{3}\\J({\sqrt {70}}i)&=\left(1+{\tfrac {9}{4}}\left(303+220{\sqrt {2}}+139{\sqrt {5}}+96{\sqrt {10}}\right)^{2}\right)^{3}\\J({\sqrt {94}}i)&=\left(1+{\tfrac {9}{8192}}\left(3+2{\sqrt {2}}+{\sqrt {9+8{\sqrt {2}}}}\right)^{8}\left(8+\left(-1-{\sqrt {2}}+{\sqrt {9+8{\sqrt {2}}}}\right)\left(-2+2{\sqrt {2}}+{\sqrt {3+4{\sqrt {2}}+3{\sqrt {9+8{\sqrt {2}}}}}}\right)\right)^{2}\right)^{3}\\J(7i)&=\left(1+{\tfrac {9}{32}}{\sqrt[{4}]{28}}\left(3+{\sqrt {7}}\right)^{3}\left(13+3{\sqrt {7}}+\left(6+{\sqrt {7}}\right){\sqrt[{4}]{28}}\right)^{2}\right)^{3}\\J(8i)&=\left(1+{\tfrac {9}{4}}{\sqrt[{4}]{2}}\left(1+{\sqrt {2}}\right)\left(123+104{\sqrt[{4}]{2}}+88{\sqrt {2}}+73{\sqrt[{4}]{8}}\right)^{2}\right)^{3}\\J(10i)&=\left(1+{\tfrac {9}{8}}\left(2402+1607{\sqrt[{4}]{5}}+1074{\sqrt[{4}]{25}}+719{\sqrt[{4}]{125}}\right)^{2}\right)^{3}\\J\left({\tfrac {5i}{2}}\right)&=\left(1+{\tfrac {9}{8}}\left(2402-1607{\sqrt[{4}]{5}}+1074{\sqrt[{4}]{25}}-719{\sqrt[{4}]{125}}\right)^{2}\right)^{3}\\J({\sqrt {130}}i)&=\left(1+{\tfrac {9}{4}}\left(7392+3289{\sqrt {5}}+2040{\sqrt {13}}+917{\sqrt {65}}\right)^{2}\right)^{3}\\J({\sqrt {190}}i)&=\left(1+18\left(31570+22323{\sqrt {2}}+14139{\sqrt {5}}+9998{\sqrt {10}}\right)^{2}\right)^{3}\\J(2{\sqrt {58}}i)&=\left(1+{\tfrac {9}{256}}\left(1+{\sqrt {2}}\right)^{5}\left(5+{\sqrt {29}}\right)^{5}\left(793+907{\sqrt {2}}+237{\sqrt {29}}+103{\sqrt {58}}\right)^{2}\right)^{3}\\J\left({\tfrac {1+{\sqrt {1435}}i}{2}}\right)&=\left(1-9\left(9892538+4424079{\sqrt {5}}+1544955{\sqrt {41}}+690925{\sqrt {205}}\right)^{2}\right)^{3}\\J\left({\tfrac {1+{\sqrt {1555}}i}{2}}\right)&=\left(1-9\left(22297077+9971556{\sqrt {5}}+\left(3571365+1597163{\sqrt {5}}\right){\sqrt {\tfrac {31+21{\sqrt {5}}}{2}}}\right)^{2}\right)^{3}\\\end{aligned}}}
2 0 1 4 年 に は い く つ か の 特 殊 値 が 計 算 さ れ た [ 8 ] 。
J
(
5 i +
2
4
)
=
(
1 −
9
(
1 +
5
)
38
2
41
2
(
7485
−
762
2
+
1479
5
−
3072
10
−
5
4
(
178
−
2221
2
+
3148
5
−
1289
10
)
)
2
)
3
J
(
10 i +
1
2
)
=
(
1 −
9
(
1 +
5
)
38
2
41
2
(
7485
−
762
2
+
1479
5
−
3072
10
+
5
4
(
178
−
2221
2
+
3148
5
−
1289
10
)
)
2
)
3
J
(
5 i
4
)
=
(
1 +
9
(
1 +
5
)
38
2
41
2
(
7485
+
762
2
+
1479
5
+
3072
10
−
5
4
(
178
+
2221
2
+
3148
5
+
1289
10
)
)
2
)
3
J (
20 i )
=
(
1 +
9
(
1 +
5
)
38
2
41
2
(
7485
+
762
2
+
1479
5
+
3072
10
+
5
4
(
178
+
2221
2
+
3148
5
+
1289
10
)
)
2
)
3
{\displaystyle {\begin{aligned}J\left({\tfrac {5i+2}{4}}\right)&=\left(1-{\tfrac {9\left(1+{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485-762{\sqrt {2}}+1479{\sqrt {5}}-3072{\sqrt {10}}-{\sqrt[{4}]{5}}\left(178-2221{\sqrt {2}}+3148{\sqrt {5}}-1289{\sqrt {10}}\right){\Bigr )}^{2}\right)^{3}\\J\left({\tfrac {10i+1}{2}}\right)&=\left(1-{\tfrac {9\left(1+{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485-762{\sqrt {2}}+1479{\sqrt {5}}-3072{\sqrt {10}}+{\sqrt[{4}]{5}}\left(178-2221{\sqrt {2}}+3148{\sqrt {5}}-1289{\sqrt {10}}\right){\Bigr )}^{2}\right)^{3}\\J\left({\tfrac {5i}{4}}\right)&=\left(1+{\tfrac {9\left(1+{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485+762{\sqrt {2}}+1479{\sqrt {5}}+3072{\sqrt {10}}-{\sqrt[{4}]{5}}\left(178+2221{\sqrt {2}}+3148{\sqrt {5}}+1289{\sqrt {10}}\right){\Big )}^{2}\right)^{3}\\J(20i)&=\left(1+{\tfrac {9\left(1+{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485+762{\sqrt {2}}+1479{\sqrt {5}}+3072{\sqrt {10}}+{\sqrt[{4}]{5}}\left(178+2221{\sqrt {2}}+3148{\sqrt {5}}+1289{\sqrt {10}}\right){\Bigr )}^{2}\right)^{3}\end{aligned}}}
こ れ 以 前 に 示 し た す べ て の 値 は 実 数 で あ る 。 複 素 共 役 の ペ ア は 、
J (
10 i )
{\displaystyle J(10i)}
と
J (
5 i
/
2 )
{\displaystyle J(5i/2)}
に 対 し 、 参 考 文 献 の よ う に 値 に 沿 っ て 、 上 記 の よ う に 対 称 的 に な っ て い る と 推 察 さ れ る 。
J
(
5 i ±
1
4
)
=
(
1 −
9 8
(
(
2402
−
1074
5
)
i ±
(
1607
−
719
5
)
5
4
)
2
)
3
{\displaystyle {\begin{aligned}J\left({\tfrac {5i\pm 1}{4}}\right)&=\left(1-{\tfrac {9}{8}}\left((2402-1074{\sqrt {5}})i\pm (1607-719{\sqrt {5}}){\sqrt[{4}]{5}}\right)^{2}\right)^{3}\end{aligned}}}
4 つ の 特 殊 値 は 、 2 つ の 複 素 共 役 の ペ ア に よ り 与 え ら れ る [ 9 ] 。
J
(
4
(
5
i
±
1
)
13
)
=
(
1
−
9
(
1
−
5
)
38
2
41
2
(
7485
−
762
2
−
1479
5
+
3072
10
±
i
5
4
(
178
−
2221
2
−
3148
5
+
1289
10
)
)
2
)
3
J
(
5
(
4
i
±
1
)
17
)
=
(
1
+
9
(
1
−
5
)
38
2
41
2
(
7485
+
762
2
−
1479
5
−
3072
10
±
i
5
4
(
178
+
2221
2
−
3148
5
−
1289
10
)
)
2
)
3
{\displaystyle {\begin{aligned}J\left({\tfrac {4\left(5i\pm 1\right)}{13}}\right)=\left(1-{\tfrac {9\left(1-{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485-762{\sqrt {2}}-1479{\sqrt {5}}+3072{\sqrt {10}}\pm i{\sqrt[{4}]{5}}\left(178-2221{\sqrt {2}}-3148{\sqrt {5}}+1289{\sqrt {10}}\right){\Bigr )}^{2}\right)^{3}\\J\left({\tfrac {5\left(4i\pm 1\right)}{17}}\right)=\left(1+{\tfrac {9\left(1-{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485+762{\sqrt {2}}-1479{\sqrt {5}}-3072{\sqrt {10}}\pm i{\sqrt[{4}]{5}}\left(178+2221{\sqrt {2}}-3148{\sqrt {5}}-1289{\sqrt {10}}\right){\Bigr )}^{2}\right)^{3}\end{aligned}}}
参考文献 [ 編集 ]
^ Silverman, Joseph H. (1986). The Arithmetic of Elliptic Curves . Graduate Texts in Mathematics . 106 . Springer-Verlag . p. 339. ISBN 0-387-96203-4 . Zbl 0585.14026 ^ Petersson, Hans (1932). Über die Entwicklungskoeffizienten der automorphen Formen . 58 . 169–215. doi :10.1007/BF02547776 . MR 1555346 ^ Rademacher, Hans (1938). The Fourier coefficients of the modular invariant j(τ) . 60 . The Johns Hopkins University Press. 501–512. doi :10.2307/2371313 . JSTOR 2371313 . MR 1507331 ^ Chandrasekharan (1985) p.108
^ Chandrasekharan, K. (1985), Elliptic Functions , Grundlehren der mathematischen Wissenschaften, 281 , Springer-Verlag , p. 110, ISBN 3-540-15295-4 , Zbl 0575.33001 ^ Girondo, Ernesto; González-Diez, Gabino (2012), Introduction to compact Riemann surfaces and dessins d'enfants , London Mathematical Society Student Texts, 79 , Cambridge: Cambridge University Press , p. 267, ISBN 978-0-521-74022-7 , Zbl 1253.30001 ^ Borcherds, R.E. (1992). Monstrous moonshine and monstrous Lie superalgebras . 60 . 405–
444. ^ Adlaj, Semjon. “Multiplication and division on elliptic curves, torsion points and roots of modular equations ”. 2014年10月17日 閲覧。 ^ Adlaj, Semjon (2014年). “Torsion points on elliptic curves and modular polynomial symmetries ”. 2014年10月15日 閲覧。
Apostol, Tom M. (1976), Modular functions and Dirichlet Series in Number Theory , Graduate Texts in Mathematics, 41 , New York: Springer-Verlag, MR 0422157 Berndt, Bruce C. ; Chan, Heng Huat (1999), “Ramanujan and the modular j-invariant” , Canadian Mathematical Bulletin 42 (4): 427–440, doi :10.4153/CMB-1999-050-1 , MR 1727340 , http://www.journals.cms.math.ca/cgi-bin/vault/public/view/berndt7376/body/PDF/berndt7376.pdf Cox, David A. (1989), Primes of the Form x^2 + ny^2: Fermat, Class Field Theory, and Complex Multiplication , New York: Wiley-Interscience Publication, John Wiley & Sons Inc., MR 1028322 Conway, John Horton ; Norton, Simon (1979), “Monstrous moonshine”, Bulletin of the London Mathematical Society 11 (3): 308–339, doi :10.1112/blms/11.3.308 , MR 0554399 Rankin, Robert A. (1977), Modular forms and functions , Cambridge: Cambridge University Press, ISBN 0-521-21212-X , MR 0498390 Schneider, Theodor (1937), “Arithmetische Untersuchungen elliptischer Integrale”, Math. Annalen 113 : 1–13, doi :10.1007/BF01571618 , MR 1513075

 jの有理函数はモジュラーであり、実際にすべてのモジュラー函数を与える。古典的には、j-不変量は C上の楕円曲線のパラメータ化として研究されていたが、驚くべきことに、モンスター群の対称性との関係を持っている︵この関係はモンストラス・ムーンシャインと呼ばれる︶。
jの有理函数はモジュラーであり、実際にすべてのモジュラー函数を与える。古典的には、j-不変量は C上の楕円曲線のパラメータ化として研究されていたが、驚くべきことに、モンスター群の対称性との関係を持っている︵この関係はモンストラス・ムーンシャインと呼ばれる︶。
 jの有理函数はモジュラーであり、実際にすべてのモジュラー函数を与える。古典的には、j-不変量は C上の楕円曲線のパラメータ化として研究されていたが、驚くべきことに、モンスター群の対称性との関係を持っている︵この関係はモンストラス・ムーンシャインと呼ばれる︶。
jの有理函数はモジュラーであり、実際にすべてのモジュラー函数を与える。古典的には、j-不変量は C上の楕円曲線のパラメータ化として研究されていたが、驚くべきことに、モンスター群の対称性との関係を持っている︵この関係はモンストラス・ムーンシャインと呼ばれる︶。

 と定義すると、この格子はヴァイエルシュトラスの楕円函数を通して、y2 = 4x3 − g2x - g3 で定義された C上の楕円曲線に対応する。このとき、j-不変量は、
と定義すると、この格子はヴァイエルシュトラスの楕円函数を通して、y2 = 4x3 − g2x - g3 で定義された C上の楕円曲線に対応する。このとき、j-不変量は、
 と定義される。ここにモジュラー判別式(modular discriminant) Δ は
と定義される。ここにモジュラー判別式(modular discriminant) Δ は
 である。
Δ はウェイト12のモジュラー形式であることと、g2 はウェイト4のモジュラー形式であるのでその3乗はウェイト12であることを示すことができる。したがって、 jがこれらの商であることから jはウェイト 0 のモジュラ函数であり、特に、SL(2, Z) の作用の下に不変な有理型函数 H→ Cである。以下に説明するように jは全射であり、このことは C上の楕円曲線の同型類と複素数の間の全単射を与えることを意味する。
である。
Δ はウェイト12のモジュラー形式であることと、g2 はウェイト4のモジュラー形式であるのでその3乗はウェイト12であることを示すことができる。したがって、 jがこれらの商であることから jはウェイト 0 のモジュラ函数であり、特に、SL(2, Z) の作用の下に不変な有理型函数 H→ Cである。以下に説明するように jは全射であり、このことは C上の楕円曲線の同型類と複素数の間の全単射を与えることを意味する。
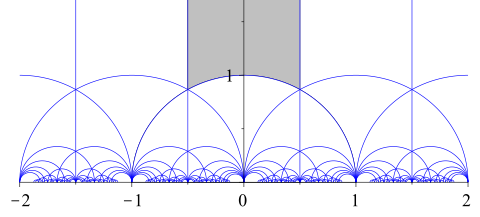
 を選択することにより、τ をjの基本領域(fundamental region)内にあり jに対して同じ値をとる、ある値に帰着させることができる。基本領域は次の条件を満たす τ から構成されている。
を選択することにより、τ をjの基本領域(fundamental region)内にあり jに対して同じ値をとる、ある値に帰着させることができる。基本領域は次の条件を満たす τ から構成されている。
 函数 j(τ) をこの領域へ制限すると、複素数 Cのすべての値をちょうど一度だけ取る。言い換えると、C すべての元 cに対し、c = j(τ) となる基本領域の元 τ が一意に存在する。このように、j は基本領域を全複素平面へ写像するという性質を持っている。
リーマン面として、基本領域の種数は 0 であり、すべての︵レベル1の︶モジュラー函数は jの有理函数であり、逆に、j のすべての有理函数はモジュラー函数である。言い換えると、モジュラー函数全体のなす体は C(j) である。
函数 j(τ) をこの領域へ制限すると、複素数 Cのすべての値をちょうど一度だけ取る。言い換えると、C すべての元 cに対し、c = j(τ) となる基本領域の元 τ が一意に存在する。このように、j は基本領域を全複素平面へ写像するという性質を持っている。
リーマン面として、基本領域の種数は 0 であり、すべての︵レベル1の︶モジュラー函数は jの有理函数であり、逆に、j のすべての有理函数はモジュラー函数である。言い換えると、モジュラー函数全体のなす体は C(j) である。

 で始まっている。
なお、jは尖点で一位の単純極を持つので、q-展開にはq−1 未満の項がない。
このフーリエ係数はすべて整数であり、このことがいくつかの概整数、例えば有名なラマヌジャン定数(Ramanujan's constant)の理由となる。
で始まっている。
なお、jは尖点で一位の単純極を持つので、q-展開にはq−1 未満の項がない。
このフーリエ係数はすべて整数であり、このことがいくつかの概整数、例えば有名なラマヌジャン定数(Ramanujan's constant)の理由となる。
 qn の係数の漸近公式(asymptotic formula)は、ハーディ・リトルウッドの円周法(Hardy–Littlewood circle method)で示すことができたように、
qn の係数の漸近公式(asymptotic formula)は、ハーディ・リトルウッドの円周法(Hardy–Littlewood circle method)で示すことができたように、
 ,
により与えられる。[2][3]
,
により与えられる。[2][3]

 と定義すると、この格子はヴァイエルシュトラスの楕円函数を通して、y2 = 4x3 − g2x - g3 で定義された C上の楕円曲線に対応する。このとき、j-不変量は、
と定義すると、この格子はヴァイエルシュトラスの楕円函数を通して、y2 = 4x3 − g2x - g3 で定義された C上の楕円曲線に対応する。このとき、j-不変量は、
 と定義される。ここにモジュラー判別式(modular discriminant) Δ は
と定義される。ここにモジュラー判別式(modular discriminant) Δ は
 である。
Δ はウェイト12のモジュラー形式であることと、g2 はウェイト4のモジュラー形式であるのでその3乗はウェイト12であることを示すことができる。したがって、 jがこれらの商であることから jはウェイト 0 のモジュラ函数であり、特に、SL(2, Z) の作用の下に不変な有理型函数 H→ Cである。以下に説明するように jは全射であり、このことは C上の楕円曲線の同型類と複素数の間の全単射を与えることを意味する。
である。
Δ はウェイト12のモジュラー形式であることと、g2 はウェイト4のモジュラー形式であるのでその3乗はウェイト12であることを示すことができる。したがって、 jがこれらの商であることから jはウェイト 0 のモジュラ函数であり、特に、SL(2, Z) の作用の下に不変な有理型函数 H→ Cである。以下に説明するように jは全射であり、このことは C上の楕円曲線の同型類と複素数の間の全単射を与えることを意味する。

 を選択することにより、τ をjの基本領域(fundamental region)内にあり jに対して同じ値をとる、ある値に帰着させることができる。基本領域は次の条件を満たす τ から構成されている。
を選択することにより、τ をjの基本領域(fundamental region)内にあり jに対して同じ値をとる、ある値に帰着させることができる。基本領域は次の条件を満たす τ から構成されている。
 函数 j(τ) をこの領域へ制限すると、複素数 Cのすべての値をちょうど一度だけ取る。言い換えると、C すべての元 cに対し、c = j(τ) となる基本領域の元 τ が一意に存在する。このように、j は基本領域を全複素平面へ写像するという性質を持っている。
リーマン面として、基本領域の種数は 0 であり、すべての︵レベル1の︶モジュラー函数は jの有理函数であり、逆に、j のすべての有理函数はモジュラー函数である。言い換えると、モジュラー函数全体のなす体は C(j) である。
函数 j(τ) をこの領域へ制限すると、複素数 Cのすべての値をちょうど一度だけ取る。言い換えると、C すべての元 cに対し、c = j(τ) となる基本領域の元 τ が一意に存在する。このように、j は基本領域を全複素平面へ写像するという性質を持っている。
リーマン面として、基本領域の種数は 0 であり、すべての︵レベル1の︶モジュラー函数は jの有理函数であり、逆に、j のすべての有理函数はモジュラー函数である。言い換えると、モジュラー函数全体のなす体は C(j) である。

 で始まっている。
なお、jは尖点で一位の単純極を持つので、q-展開にはq−1 未満の項がない。
このフーリエ係数はすべて整数であり、このことがいくつかの概整数、例えば有名なラマヌジャン定数(Ramanujan's constant)の理由となる。
で始まっている。
なお、jは尖点で一位の単純極を持つので、q-展開にはq−1 未満の項がない。
このフーリエ係数はすべて整数であり、このことがいくつかの概整数、例えば有名なラマヌジャン定数(Ramanujan's constant)の理由となる。
 qn の係数の漸近公式(asymptotic formula)は、ハーディ・リトルウッドの円周法(Hardy–Littlewood circle method)で示すことができたように、
qn の係数の漸近公式(asymptotic formula)は、ハーディ・リトルウッドの円周法(Hardy–Littlewood circle method)で示すことができたように、
 ,
により与えられる。[2][3]
,
により与えられる。[2][3]
 という形式に正規化される種数 0 のモジュラ函数が、有限個しか存在しないことを証明した。
という形式に正規化される種数 0 のモジュラ函数が、有限個しか存在しないことを証明した。
 を得る。
を得る。
 は、ヤコビのテータ函数
は、ヤコビのテータ函数  の比率であり、楕円モジュラス
の比率であり、楕円モジュラス  の二乗である。[4] λ が次の非調和比(cross-ratio)の6つの値で入れ替わるときは、jの値は不変である[5]。
の二乗である。[4] λ が次の非調和比(cross-ratio)の6つの値で入れ替わるときは、jの値は不変である[5]。
 jの分岐点は {0, 1, ∞} であるので、ベリイ函数(Belyi function)である[6]。
jの分岐点は {0, 1, ∞} であるので、ベリイ函数(Belyi function)である[6]。
 ︵ノーム︶と定義し直すと、ヤコビのテータ函数
︵ノーム︶と定義し直すと、ヤコビのテータ函数
 から指標付きテータ函数を導くことができる。次のように置くこととする。
から指標付きテータ函数を導くことができる。次のように置くこととする。


 ここに
ここに  と
と  は記法を変えたものとした。すると、ヴァイエルシュトラス定数g2, g3 とデデキントのエータ函数 η(τ) に対して、
は記法を変えたものとした。すると、ヴァイエルシュトラス定数g2, g3 とデデキントのエータ函数 η(τ) に対して、


 となる。このようにすると、j (τ) を早く計算できる形に書き換えることができる。
となる。このようにすると、j (τ) を早く計算できる形に書き換えることができる。
 ただし、
ただし、
 であることに注意する。
であることに注意する。
 を任意の体の上の平面楕円曲線とすると、
を任意の体の上の平面楕円曲線とすると、


 と定義することができ、
と定義することができ、
 と表すと、これは楕円曲線の判別式を表している。
ここで、楕円曲線の j-不変量を
と表すと、これは楕円曲線の判別式を表している。
ここで、楕円曲線の j-不変量を
 と定義する。
楕円曲線が定義されている体の標数が2もしくは3でない場合に、この定義は
と定義する。
楕円曲線が定義されている体の標数が2もしくは3でない場合に、この定義は
 と書き直すことができる。
と書き直すことができる。
 x = λ(1−λ) とすると6次式はxの3次式となる。すると、λ の6つの値のどれに対しても、
x = λ(1−λ) とすると6次式はxの3次式となる。すると、λ の6つの値のどれに対しても、
 となる。
方法2: γ の4次式を解く方法。
となる。
方法2: γ の4次式を解く方法。
 任意の4つの根に対して、
任意の4つの根に対して、
 となる。
方法3: β の3次式を解く方法。
となる。
方法3: β の3次式を解く方法。
 すると、任意の3つの根に対し、
すると、任意の3つの根に対し、
 となる。
方法4: α の2次式を解く方法。
となる。
方法4: α の2次式を解く方法。
 すると、
すると、
 となる。
2つの根は τ と -1/τ であるが、j (τ) = j (-1/τ) であるために、どの α を選んでも差異はない。後半3つの方法は、ラマヌジャンの交代基底についての楕円函数論で発見された。
逆函数は、これらの根の比率が有界でないにもかかわらず、楕円函数の周期の高精度な計算を通して、うまく適用することが可能である。また、関連する帰結として、2のべきの大きさをもつ虚数軸上の点でjの値が二次の根となることを通して︵逆関数を︶表すことができる︵このようにして、定規とコンパスによる作図が可能となる︶。レベルが2のモジュラ函数は3次式であるので、この結果は自明ではない。
となる。
2つの根は τ と -1/τ であるが、j (τ) = j (-1/τ) であるために、どの α を選んでも差異はない。後半3つの方法は、ラマヌジャンの交代基底についての楕円函数論で発見された。
逆函数は、これらの根の比率が有界でないにもかかわらず、楕円函数の周期の高精度な計算を通して、うまく適用することが可能である。また、関連する帰結として、2のべきの大きさをもつ虚数軸上の点でjの値が二次の根となることを通して︵逆関数を︶表すことができる︵このようにして、定規とコンパスによる作図が可能となる︶。レベルが2のモジュラ函数は3次式であるので、この結果は自明ではない。
 を発見し、
を発見し、 という事実を示すことに使用した。同様な公式は、ラマヌジャン・佐藤級数(Ramanujan-Sato series)を参照。
という事実を示すことに使用した。同様な公式は、ラマヌジャン・佐藤級数(Ramanujan-Sato series)を参照。
 である︵ここでc_nはj関数のq展開におけるq^nの係数︶.
である︵ここでc_nはj関数のq展開におけるq^nの係数︶.
 では 0 となる。
以下に、いくつかの特殊値を示す︵J = j/1728 を使って表示している︶[疑問点]。
では 0 となる。
以下に、いくつかの特殊値を示す︵J = j/1728 を使って表示している︶[疑問点]。
![{\displaystyle {\begin{aligned}J(i)&=J\left({\tfrac {1+i}{2}}\right)=1\\J\left({\sqrt {2}}i\right)&={\big (}{\tfrac {5}{3}}{\big )}^{3}\\J(2i)&={\big (}{\tfrac {11}{2}}{\big )}^{3}\\J\left(2{\sqrt {2}}i\right)&={\tfrac {125}{216}}\left(19+13{\sqrt {2}}\right)^{3}\\J(4i)&={\tfrac {1}{64}}\left(724+513{\sqrt {2}}\right)^{3}\\J\left({\tfrac {1+2i}{2}}\right)&={\tfrac {1}{64}}\left(724-513{\sqrt {2}}\right)^{3}\\J\left({\tfrac {1+2{\sqrt {2}}i}{3}}\right)&={\tfrac {125}{216}}\left(19-13{\sqrt {2}}\right)^{3}\\J(3i)&={\tfrac {1}{27}}\left(2+{\sqrt {3}}\right)^{2}\left(21+20{\sqrt {3}}\right)^{3}\\J\left(2{\sqrt {3}}i\right)&={\tfrac {125}{16}}\left(30+17{\sqrt {3}}\right)^{3}\\J\left({\tfrac {1+7{\sqrt {3}}i}{2}}\right)&=-{\tfrac {64000}{7}}\left(651+142{\sqrt {21}}\right)^{3}\\J\left({\tfrac {1+3{\sqrt {11}}i}{10}}\right)&={\tfrac {64}{27}}\left(23-4{\sqrt {33}}\right)^{2}\left(-77+15{\sqrt {33}}\right)^{3}\\J\left({\sqrt {21}}i\right)&={\tfrac {1}{128}}\left(3+{\sqrt {7}}\right)^{5}\left(17+7{\sqrt {3}}+59{\sqrt {7}}+35{\sqrt {21}}\right)^{3}\\J\left({\tfrac {{\sqrt {30}}i}{1}}\right)&={\tfrac {1}{4}}\left(7+5{\sqrt {2}}+3{\sqrt {5}}+2{\sqrt {10}}\right)^{4}\left(55+30{\sqrt {2}}+12{\sqrt {5}}+10{\sqrt {10}}\right)^{3}\\J\left({\tfrac {{\sqrt {30}}i}{2}}\right)&={\tfrac {1}{4}}\left(7+5{\sqrt {2}}-3{\sqrt {5}}-2{\sqrt {10}}\right)^{4}\left(55+30{\sqrt {2}}-12{\sqrt {5}}-10{\sqrt {10}}\right)^{3}\\J\left({\tfrac {{\sqrt {30}}i}{5}}\right)&={\tfrac {1}{4}}\left(7-5{\sqrt {2}}+3{\sqrt {5}}-2{\sqrt {10}}\right)^{4}\left(55-30{\sqrt {2}}+12{\sqrt {5}}-10{\sqrt {10}}\right)^{3}\\J\left({\tfrac {{\sqrt {30}}i}{10}}\right)&={\tfrac {1}{4}}\left(7-5{\sqrt {2}}-3{\sqrt {5}}+2{\sqrt {10}}\right)^{4}\left(55-30{\sqrt {2}}-12{\sqrt {5}}+10{\sqrt {10}}\right)^{3}\\J\left({\tfrac {1+{\sqrt {31}}i}{2}}\right)&=\left(1-\left(1+{\frac {\sqrt {19}}{2}}\left({\sqrt {\tfrac {13-{\sqrt {93}}}{13+{\sqrt {93}}}}}\cdot {\sqrt[{3}]{\tfrac {{\sqrt {31}}+{\sqrt {27}}}{{\sqrt {31}}-{\sqrt {27}}}}}+{\sqrt {\tfrac {13+{\sqrt {93}}}{13-{\sqrt {93}}}}}\cdot {\sqrt[{3}]{\tfrac {{\sqrt {31}}-{\sqrt {27}}}{{\sqrt {31}}+{\sqrt {27}}}}}\right)\right)^{2}\right)^{3}\\J(5i)&=\left(1+{\tfrac {9}{4}}{\sqrt {5}}\left(13+5{\sqrt {5}}\right)^{2}\right)^{3}\\J\left({\tfrac {5i+1}{2}}\right)&=\left(1-{\tfrac {9}{4}}{\sqrt {5}}\left(13-5{\sqrt {5}}\right)^{2}\right)^{3}\\J(6i)&={\tfrac {1}{216}}\left(2+{\sqrt {3}}\right)^{10}\left(231+380{\sqrt {3}}+\left(204+158{\sqrt {3}}\right){\sqrt[{4}]{12}}\right)^{3}\\J({\sqrt {70}}i)&=\left(1+{\tfrac {9}{4}}\left(303+220{\sqrt {2}}+139{\sqrt {5}}+96{\sqrt {10}}\right)^{2}\right)^{3}\\J({\sqrt {94}}i)&=\left(1+{\tfrac {9}{8192}}\left(3+2{\sqrt {2}}+{\sqrt {9+8{\sqrt {2}}}}\right)^{8}\left(8+\left(-1-{\sqrt {2}}+{\sqrt {9+8{\sqrt {2}}}}\right)\left(-2+2{\sqrt {2}}+{\sqrt {3+4{\sqrt {2}}+3{\sqrt {9+8{\sqrt {2}}}}}}\right)\right)^{2}\right)^{3}\\J(7i)&=\left(1+{\tfrac {9}{32}}{\sqrt[{4}]{28}}\left(3+{\sqrt {7}}\right)^{3}\left(13+3{\sqrt {7}}+\left(6+{\sqrt {7}}\right){\sqrt[{4}]{28}}\right)^{2}\right)^{3}\\J(8i)&=\left(1+{\tfrac {9}{4}}{\sqrt[{4}]{2}}\left(1+{\sqrt {2}}\right)\left(123+104{\sqrt[{4}]{2}}+88{\sqrt {2}}+73{\sqrt[{4}]{8}}\right)^{2}\right)^{3}\\J(10i)&=\left(1+{\tfrac {9}{8}}\left(2402+1607{\sqrt[{4}]{5}}+1074{\sqrt[{4}]{25}}+719{\sqrt[{4}]{125}}\right)^{2}\right)^{3}\\J\left({\tfrac {5i}{2}}\right)&=\left(1+{\tfrac {9}{8}}\left(2402-1607{\sqrt[{4}]{5}}+1074{\sqrt[{4}]{25}}-719{\sqrt[{4}]{125}}\right)^{2}\right)^{3}\\J({\sqrt {130}}i)&=\left(1+{\tfrac {9}{4}}\left(7392+3289{\sqrt {5}}+2040{\sqrt {13}}+917{\sqrt {65}}\right)^{2}\right)^{3}\\J({\sqrt {190}}i)&=\left(1+18\left(31570+22323{\sqrt {2}}+14139{\sqrt {5}}+9998{\sqrt {10}}\right)^{2}\right)^{3}\\J(2{\sqrt {58}}i)&=\left(1+{\tfrac {9}{256}}\left(1+{\sqrt {2}}\right)^{5}\left(5+{\sqrt {29}}\right)^{5}\left(793+907{\sqrt {2}}+237{\sqrt {29}}+103{\sqrt {58}}\right)^{2}\right)^{3}\\J\left({\tfrac {1+{\sqrt {1435}}i}{2}}\right)&=\left(1-9\left(9892538+4424079{\sqrt {5}}+1544955{\sqrt {41}}+690925{\sqrt {205}}\right)^{2}\right)^{3}\\J\left({\tfrac {1+{\sqrt {1555}}i}{2}}\right)&=\left(1-9\left(22297077+9971556{\sqrt {5}}+\left(3571365+1597163{\sqrt {5}}\right){\sqrt {\tfrac {31+21{\sqrt {5}}}{2}}}\right)^{2}\right)^{3}\\\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/969a1013599eecc294a857391fee5d3eba91ed6f) 2014年にはいくつかの特殊値が計算された[8]。
2014年にはいくつかの特殊値が計算された[8]。
![{\displaystyle {\begin{aligned}J\left({\tfrac {5i+2}{4}}\right)&=\left(1-{\tfrac {9\left(1+{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485-762{\sqrt {2}}+1479{\sqrt {5}}-3072{\sqrt {10}}-{\sqrt[{4}]{5}}\left(178-2221{\sqrt {2}}+3148{\sqrt {5}}-1289{\sqrt {10}}\right){\Bigr )}^{2}\right)^{3}\\J\left({\tfrac {10i+1}{2}}\right)&=\left(1-{\tfrac {9\left(1+{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485-762{\sqrt {2}}+1479{\sqrt {5}}-3072{\sqrt {10}}+{\sqrt[{4}]{5}}\left(178-2221{\sqrt {2}}+3148{\sqrt {5}}-1289{\sqrt {10}}\right){\Bigr )}^{2}\right)^{3}\\J\left({\tfrac {5i}{4}}\right)&=\left(1+{\tfrac {9\left(1+{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485+762{\sqrt {2}}+1479{\sqrt {5}}+3072{\sqrt {10}}-{\sqrt[{4}]{5}}\left(178+2221{\sqrt {2}}+3148{\sqrt {5}}+1289{\sqrt {10}}\right){\Big )}^{2}\right)^{3}\\J(20i)&=\left(1+{\tfrac {9\left(1+{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485+762{\sqrt {2}}+1479{\sqrt {5}}+3072{\sqrt {10}}+{\sqrt[{4}]{5}}\left(178+2221{\sqrt {2}}+3148{\sqrt {5}}+1289{\sqrt {10}}\right){\Bigr )}^{2}\right)^{3}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3bba1c724ceb674a5a3f4a7c5831fea7b9734e6d) これ以前に示したすべての値は実数である。複素共役のペアは、
これ以前に示したすべての値は実数である。複素共役のペアは、 と
と  に対し、参考文献のように値に沿って、上記のように対称的になっていると推察される。
に対し、参考文献のように値に沿って、上記のように対称的になっていると推察される。
![{\displaystyle {\begin{aligned}J\left({\tfrac {5i\pm 1}{4}}\right)&=\left(1-{\tfrac {9}{8}}\left((2402-1074{\sqrt {5}})i\pm (1607-719{\sqrt {5}}){\sqrt[{4}]{5}}\right)^{2}\right)^{3}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5566614a3b8aca7521b71e8d9068b85a85f1a52b) 4つの特殊値は、2つの複素共役のペアにより与えられる[9]。
4つの特殊値は、2つの複素共役のペアにより与えられる[9]。
 という形式に正規化される種数 0 のモジュラ函数が、有限個しか存在しないことを証明した。
という形式に正規化される種数 0 のモジュラ函数が、有限個しか存在しないことを証明した。
 を得る。
を得る。
 は、ヤコビのテータ函数
は、ヤコビのテータ函数  の比率であり、楕円モジュラス
の比率であり、楕円モジュラス  の二乗である。[4] λ が次の非調和比(cross-ratio)の6つの値で入れ替わるときは、jの値は不変である[5]。
の二乗である。[4] λ が次の非調和比(cross-ratio)の6つの値で入れ替わるときは、jの値は不変である[5]。
 jの分岐点は {0, 1, ∞} であるので、ベリイ函数(Belyi function)である[6]。
jの分岐点は {0, 1, ∞} であるので、ベリイ函数(Belyi function)である[6]。
 ︵ノーム︶と定義し直すと、ヤコビのテータ函数
︵ノーム︶と定義し直すと、ヤコビのテータ函数
 から指標付きテータ函数を導くことができる。次のように置くこととする。
から指標付きテータ函数を導くことができる。次のように置くこととする。


 ここに
ここに  と
と  は記法を変えたものとした。すると、ヴァイエルシュトラス定数g2, g3 とデデキントのエータ函数 η(τ) に対して、
は記法を変えたものとした。すると、ヴァイエルシュトラス定数g2, g3 とデデキントのエータ函数 η(τ) に対して、


 となる。このようにすると、j (τ) を早く計算できる形に書き換えることができる。
となる。このようにすると、j (τ) を早く計算できる形に書き換えることができる。
 ただし、
ただし、
 であることに注意する。
であることに注意する。
 を任意の体の上の平面楕円曲線とすると、
を任意の体の上の平面楕円曲線とすると、


 と定義することができ、
と定義することができ、
 と表すと、これは楕円曲線の判別式を表している。
ここで、楕円曲線の j-不変量を
と表すと、これは楕円曲線の判別式を表している。
ここで、楕円曲線の j-不変量を
 と定義する。
楕円曲線が定義されている体の標数が2もしくは3でない場合に、この定義は
と定義する。
楕円曲線が定義されている体の標数が2もしくは3でない場合に、この定義は
 と書き直すことができる。
と書き直すことができる。
 x = λ(1−λ) とすると6次式はxの3次式となる。すると、λ の6つの値のどれに対しても、
x = λ(1−λ) とすると6次式はxの3次式となる。すると、λ の6つの値のどれに対しても、
 となる。
方法2: γ の4次式を解く方法。
となる。
方法2: γ の4次式を解く方法。
 任意の4つの根に対して、
任意の4つの根に対して、
 となる。
方法3: β の3次式を解く方法。
となる。
方法3: β の3次式を解く方法。
 すると、任意の3つの根に対し、
すると、任意の3つの根に対し、
 となる。
方法4: α の2次式を解く方法。
となる。
方法4: α の2次式を解く方法。
 すると、
すると、
 となる。
2つの根は τ と -1/τ であるが、j (τ) = j (-1/τ) であるために、どの α を選んでも差異はない。後半3つの方法は、ラマヌジャンの交代基底についての楕円函数論で発見された。
逆函数は、これらの根の比率が有界でないにもかかわらず、楕円函数の周期の高精度な計算を通して、うまく適用することが可能である。また、関連する帰結として、2のべきの大きさをもつ虚数軸上の点でjの値が二次の根となることを通して︵逆関数を︶表すことができる︵このようにして、定規とコンパスによる作図が可能となる︶。レベルが2のモジュラ函数は3次式であるので、この結果は自明ではない。
となる。
2つの根は τ と -1/τ であるが、j (τ) = j (-1/τ) であるために、どの α を選んでも差異はない。後半3つの方法は、ラマヌジャンの交代基底についての楕円函数論で発見された。
逆函数は、これらの根の比率が有界でないにもかかわらず、楕円函数の周期の高精度な計算を通して、うまく適用することが可能である。また、関連する帰結として、2のべきの大きさをもつ虚数軸上の点でjの値が二次の根となることを通して︵逆関数を︶表すことができる︵このようにして、定規とコンパスによる作図が可能となる︶。レベルが2のモジュラ函数は3次式であるので、この結果は自明ではない。
 を発見し、
を発見し、 という事実を示すことに使用した。同様な公式は、ラマヌジャン・佐藤級数(Ramanujan-Sato series)を参照。
という事実を示すことに使用した。同様な公式は、ラマヌジャン・佐藤級数(Ramanujan-Sato series)を参照。
 である︵ここでc_nはj関数のq展開におけるq^nの係数︶.
である︵ここでc_nはj関数のq展開におけるq^nの係数︶.
 では 0 となる。
以下に、いくつかの特殊値を示す︵J = j/1728 を使って表示している︶[疑問点]。
では 0 となる。
以下に、いくつかの特殊値を示す︵J = j/1728 を使って表示している︶[疑問点]。
![{\displaystyle {\begin{aligned}J(i)&=J\left({\tfrac {1+i}{2}}\right)=1\\J\left({\sqrt {2}}i\right)&={\big (}{\tfrac {5}{3}}{\big )}^{3}\\J(2i)&={\big (}{\tfrac {11}{2}}{\big )}^{3}\\J\left(2{\sqrt {2}}i\right)&={\tfrac {125}{216}}\left(19+13{\sqrt {2}}\right)^{3}\\J(4i)&={\tfrac {1}{64}}\left(724+513{\sqrt {2}}\right)^{3}\\J\left({\tfrac {1+2i}{2}}\right)&={\tfrac {1}{64}}\left(724-513{\sqrt {2}}\right)^{3}\\J\left({\tfrac {1+2{\sqrt {2}}i}{3}}\right)&={\tfrac {125}{216}}\left(19-13{\sqrt {2}}\right)^{3}\\J(3i)&={\tfrac {1}{27}}\left(2+{\sqrt {3}}\right)^{2}\left(21+20{\sqrt {3}}\right)^{3}\\J\left(2{\sqrt {3}}i\right)&={\tfrac {125}{16}}\left(30+17{\sqrt {3}}\right)^{3}\\J\left({\tfrac {1+7{\sqrt {3}}i}{2}}\right)&=-{\tfrac {64000}{7}}\left(651+142{\sqrt {21}}\right)^{3}\\J\left({\tfrac {1+3{\sqrt {11}}i}{10}}\right)&={\tfrac {64}{27}}\left(23-4{\sqrt {33}}\right)^{2}\left(-77+15{\sqrt {33}}\right)^{3}\\J\left({\sqrt {21}}i\right)&={\tfrac {1}{128}}\left(3+{\sqrt {7}}\right)^{5}\left(17+7{\sqrt {3}}+59{\sqrt {7}}+35{\sqrt {21}}\right)^{3}\\J\left({\tfrac {{\sqrt {30}}i}{1}}\right)&={\tfrac {1}{4}}\left(7+5{\sqrt {2}}+3{\sqrt {5}}+2{\sqrt {10}}\right)^{4}\left(55+30{\sqrt {2}}+12{\sqrt {5}}+10{\sqrt {10}}\right)^{3}\\J\left({\tfrac {{\sqrt {30}}i}{2}}\right)&={\tfrac {1}{4}}\left(7+5{\sqrt {2}}-3{\sqrt {5}}-2{\sqrt {10}}\right)^{4}\left(55+30{\sqrt {2}}-12{\sqrt {5}}-10{\sqrt {10}}\right)^{3}\\J\left({\tfrac {{\sqrt {30}}i}{5}}\right)&={\tfrac {1}{4}}\left(7-5{\sqrt {2}}+3{\sqrt {5}}-2{\sqrt {10}}\right)^{4}\left(55-30{\sqrt {2}}+12{\sqrt {5}}-10{\sqrt {10}}\right)^{3}\\J\left({\tfrac {{\sqrt {30}}i}{10}}\right)&={\tfrac {1}{4}}\left(7-5{\sqrt {2}}-3{\sqrt {5}}+2{\sqrt {10}}\right)^{4}\left(55-30{\sqrt {2}}-12{\sqrt {5}}+10{\sqrt {10}}\right)^{3}\\J\left({\tfrac {1+{\sqrt {31}}i}{2}}\right)&=\left(1-\left(1+{\frac {\sqrt {19}}{2}}\left({\sqrt {\tfrac {13-{\sqrt {93}}}{13+{\sqrt {93}}}}}\cdot {\sqrt[{3}]{\tfrac {{\sqrt {31}}+{\sqrt {27}}}{{\sqrt {31}}-{\sqrt {27}}}}}+{\sqrt {\tfrac {13+{\sqrt {93}}}{13-{\sqrt {93}}}}}\cdot {\sqrt[{3}]{\tfrac {{\sqrt {31}}-{\sqrt {27}}}{{\sqrt {31}}+{\sqrt {27}}}}}\right)\right)^{2}\right)^{3}\\J(5i)&=\left(1+{\tfrac {9}{4}}{\sqrt {5}}\left(13+5{\sqrt {5}}\right)^{2}\right)^{3}\\J\left({\tfrac {5i+1}{2}}\right)&=\left(1-{\tfrac {9}{4}}{\sqrt {5}}\left(13-5{\sqrt {5}}\right)^{2}\right)^{3}\\J(6i)&={\tfrac {1}{216}}\left(2+{\sqrt {3}}\right)^{10}\left(231+380{\sqrt {3}}+\left(204+158{\sqrt {3}}\right){\sqrt[{4}]{12}}\right)^{3}\\J({\sqrt {70}}i)&=\left(1+{\tfrac {9}{4}}\left(303+220{\sqrt {2}}+139{\sqrt {5}}+96{\sqrt {10}}\right)^{2}\right)^{3}\\J({\sqrt {94}}i)&=\left(1+{\tfrac {9}{8192}}\left(3+2{\sqrt {2}}+{\sqrt {9+8{\sqrt {2}}}}\right)^{8}\left(8+\left(-1-{\sqrt {2}}+{\sqrt {9+8{\sqrt {2}}}}\right)\left(-2+2{\sqrt {2}}+{\sqrt {3+4{\sqrt {2}}+3{\sqrt {9+8{\sqrt {2}}}}}}\right)\right)^{2}\right)^{3}\\J(7i)&=\left(1+{\tfrac {9}{32}}{\sqrt[{4}]{28}}\left(3+{\sqrt {7}}\right)^{3}\left(13+3{\sqrt {7}}+\left(6+{\sqrt {7}}\right){\sqrt[{4}]{28}}\right)^{2}\right)^{3}\\J(8i)&=\left(1+{\tfrac {9}{4}}{\sqrt[{4}]{2}}\left(1+{\sqrt {2}}\right)\left(123+104{\sqrt[{4}]{2}}+88{\sqrt {2}}+73{\sqrt[{4}]{8}}\right)^{2}\right)^{3}\\J(10i)&=\left(1+{\tfrac {9}{8}}\left(2402+1607{\sqrt[{4}]{5}}+1074{\sqrt[{4}]{25}}+719{\sqrt[{4}]{125}}\right)^{2}\right)^{3}\\J\left({\tfrac {5i}{2}}\right)&=\left(1+{\tfrac {9}{8}}\left(2402-1607{\sqrt[{4}]{5}}+1074{\sqrt[{4}]{25}}-719{\sqrt[{4}]{125}}\right)^{2}\right)^{3}\\J({\sqrt {130}}i)&=\left(1+{\tfrac {9}{4}}\left(7392+3289{\sqrt {5}}+2040{\sqrt {13}}+917{\sqrt {65}}\right)^{2}\right)^{3}\\J({\sqrt {190}}i)&=\left(1+18\left(31570+22323{\sqrt {2}}+14139{\sqrt {5}}+9998{\sqrt {10}}\right)^{2}\right)^{3}\\J(2{\sqrt {58}}i)&=\left(1+{\tfrac {9}{256}}\left(1+{\sqrt {2}}\right)^{5}\left(5+{\sqrt {29}}\right)^{5}\left(793+907{\sqrt {2}}+237{\sqrt {29}}+103{\sqrt {58}}\right)^{2}\right)^{3}\\J\left({\tfrac {1+{\sqrt {1435}}i}{2}}\right)&=\left(1-9\left(9892538+4424079{\sqrt {5}}+1544955{\sqrt {41}}+690925{\sqrt {205}}\right)^{2}\right)^{3}\\J\left({\tfrac {1+{\sqrt {1555}}i}{2}}\right)&=\left(1-9\left(22297077+9971556{\sqrt {5}}+\left(3571365+1597163{\sqrt {5}}\right){\sqrt {\tfrac {31+21{\sqrt {5}}}{2}}}\right)^{2}\right)^{3}\\\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/969a1013599eecc294a857391fee5d3eba91ed6f) 2014年にはいくつかの特殊値が計算された[8]。
2014年にはいくつかの特殊値が計算された[8]。
![{\displaystyle {\begin{aligned}J\left({\tfrac {5i+2}{4}}\right)&=\left(1-{\tfrac {9\left(1+{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485-762{\sqrt {2}}+1479{\sqrt {5}}-3072{\sqrt {10}}-{\sqrt[{4}]{5}}\left(178-2221{\sqrt {2}}+3148{\sqrt {5}}-1289{\sqrt {10}}\right){\Bigr )}^{2}\right)^{3}\\J\left({\tfrac {10i+1}{2}}\right)&=\left(1-{\tfrac {9\left(1+{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485-762{\sqrt {2}}+1479{\sqrt {5}}-3072{\sqrt {10}}+{\sqrt[{4}]{5}}\left(178-2221{\sqrt {2}}+3148{\sqrt {5}}-1289{\sqrt {10}}\right){\Bigr )}^{2}\right)^{3}\\J\left({\tfrac {5i}{4}}\right)&=\left(1+{\tfrac {9\left(1+{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485+762{\sqrt {2}}+1479{\sqrt {5}}+3072{\sqrt {10}}-{\sqrt[{4}]{5}}\left(178+2221{\sqrt {2}}+3148{\sqrt {5}}+1289{\sqrt {10}}\right){\Big )}^{2}\right)^{3}\\J(20i)&=\left(1+{\tfrac {9\left(1+{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485+762{\sqrt {2}}+1479{\sqrt {5}}+3072{\sqrt {10}}+{\sqrt[{4}]{5}}\left(178+2221{\sqrt {2}}+3148{\sqrt {5}}+1289{\sqrt {10}}\right){\Bigr )}^{2}\right)^{3}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/3bba1c724ceb674a5a3f4a7c5831fea7b9734e6d) これ以前に示したすべての値は実数である。複素共役のペアは、
これ以前に示したすべての値は実数である。複素共役のペアは、 と
と  に対し、参考文献のように値に沿って、上記のように対称的になっていると推察される。
に対し、参考文献のように値に沿って、上記のように対称的になっていると推察される。
![{\displaystyle {\begin{aligned}J\left({\tfrac {5i\pm 1}{4}}\right)&=\left(1-{\tfrac {9}{8}}\left((2402-1074{\sqrt {5}})i\pm (1607-719{\sqrt {5}}){\sqrt[{4}]{5}}\right)^{2}\right)^{3}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/5566614a3b8aca7521b71e8d9068b85a85f1a52b) 4つの特殊値は、2つの複素共役のペアにより与えられる[9]。
4つの特殊値は、2つの複素共役のペアにより与えられる[9]。

![{\displaystyle {\begin{aligned}J\left({\tfrac {4\left(5i\pm 1\right)}{13}}\right)=\left(1-{\tfrac {9\left(1-{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485-762{\sqrt {2}}-1479{\sqrt {5}}+3072{\sqrt {10}}\pm i{\sqrt[{4}]{5}}\left(178-2221{\sqrt {2}}-3148{\sqrt {5}}+1289{\sqrt {10}}\right){\Bigr )}^{2}\right)^{3}\\J\left({\tfrac {5\left(4i\pm 1\right)}{17}}\right)=\left(1+{\tfrac {9\left(1-{\sqrt {5}}\right)^{38}}{2^{41}{\sqrt {2}}}}{\Bigl (}7485+762{\sqrt {2}}-1479{\sqrt {5}}-3072{\sqrt {10}}\pm i{\sqrt[{4}]{5}}\left(178+2221{\sqrt {2}}-3148{\sqrt {5}}-1289{\sqrt {10}}\right){\Bigr )}^{2}\right)^{3}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7fb9310e34bda283dfbb8bbaf8c4e252ed771a0f)