群作用

数学における群作用︵ぐんさよう、英: group action︶は、群を用いて対象の対称性を記述する方法である。

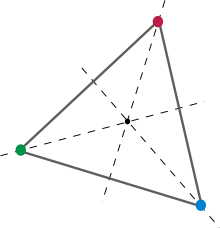
正三角形が与えられたとき、三角形の重心を中心とする反時計回りの 120° 回転は、三角形の各頂点を別な頂点に移す写像として三角形の頂点集合の上に作用する。

導入

物体の本質的な要素を集合によって表し、物体の対称性をその集合上の対称性の群︵その集合の全単射な変換からなる群︶によって記述するとき、この群は︵特に集合が有限集合であるとき︶置換群 (permutation group) あるいは︵特に集合がベクトル空間で、群作用が線型変換などであるとき︶変換群 (transformation group) と呼ばれる。

群作用は、群の各元がある集合上の全単射な変換︵対称変換︶の如く﹁作用﹂するけれども、それがそのような変換と同一視される必要は無いという点において、対称性の群の柔軟な一般化となっている。これにより、物体の対称性のより包括的な記述が可能になる。これはたとえば多面体に対して、その頂点全体の成す集合、辺全体の成す集合、面の成す集合といったいくつかの異なる集合に同じ群を作用させることによって得られる。

G が群で Xが集合であるとき、群作用は Gから Xの対称群への群準同型として定義することができる。この作用は群 Gの各元に対して Xの置換を以下のように割り当てる。

●群 Gの単位元に対応する X上の置換は、X 上の恒等変換である。

●群 Gにおけるふたつの元の積 ghに対応する X上の置換は、g および hにそれぞれ対応する置換の合成である。

ここでは Gの各元が置換として表現されているので、このような群作用は群の置換表現 (permutation representation) としても知られる。

群作用を考えることによって得られる抽象化は、幾何学的な考え方をより抽象的な対象にも応用できるという面で非常に強力である。多くの数学的対象はその上で定義される自然な群作用というものを持っており、特に群は別な群や自分自身への群作用を考えることができる。このような一般性を持つにもかかわらず、群作用の理論は︵軌道-安定化群定理 (orbit stabilizer theorem) のような︶適用範囲の広い定理を含み、さまざまな分野での深い結果を示すのに用いられる。

定義

G を群、X を集合とするとき、G の Xへの左群作用 (left group action) とは、外部二項演算

${\displaystyle L\colon G\times X\to X;\quad (g,x)\mapsto L(g,x)=:g\bullet x=L_{g}($x)}  

で、以下の二つの公理

(一)G の任意の元 g, hおよび Xの任意の元 xに対して (gh)• x= g•(h • x) が成り立つ

(二)G の単位元 eと Xの任意の元 xに対して、e • x= xが成り立つ

を満たすものを言う。このとき、集合 Xは左 G-集合 (left G-set) と呼ばれ、また群 Gは Xに︵左から︶作用 (act) するという。紛れの虞が無いならば g• xなどの演算を省略して gxのようにしばしば略記する。

二つの公理から、G の各元 gに対して x∈ Xを g• xへ写す写像は Xから Xへの全単射となることが従う︵逆写像が xを g⁻¹• xに写す写像によって与えられる︶。したがって、群 Gの Xへの作用を、群 Gから X上の全単射全体の成す対称群 Sym(X) への群準同型として定義することもできる。

まったく同様に、群 Gの集合 Xへの右群作用 (right group action) を写像 R: X× G→ X; (x, g) ↦ R(x, g) =: x• gと二つの公理

(一)x •(gh) = (x • g)• h

(二)x• e= x

によって定義することができる。右作用と左作用の違いは、gh のような積の xへの作用の順番であり、左作用ならば hを先に作用させてから gが作用するが、右作用では gが先に作用してから hが作用する。右作用に群の反転演算を合わせれば左作用が得られる。実際、R が右作用ならば

${\displaystyle G\times X\to X;\quad (g,x)\mapsto R(x,g^{-1})=R_{g}($x)}  

は左作用である。これは

${\displaystyle {\begin{aligned}R_{gh}($x)&:=R(x,(gh)^{-1})=x\bullet (h^{-1}g^{-1})\\&\,=(x\bullet h^{-1})\bullet g^{-1}=R_{h}(x)\bullet g^{-1}=(R_{g}\circ R_{h})(x),\\R_{e}(x)&:=R(x,e^{-1})=x\bullet e=x\end{aligned}}}  

から確認できる。同様に任意の左作用を右作用にすることもできる。したがって、右作用を考えることで新しく得られるものは特に無いため、理論上は左群作用のみを主に考え、これを単に群作用と称する。

例

• 任意の群 G に対して自明な作用 (trivial action) は、群 G 全体が X 上の恒等変換を誘導する、つまり G の任意の元 g と X の任意の元に対して g • x = x が成立することをいう。
• 任意の群 G は G 自身への自然だが本質的に異なる二種類の作用
  
  g • x= gx(∀x ∈ G)
  
  g • x= gxg⁻¹ (∀x ∈ G)
  を持つ。後者の作用は内部自己同型による作用、両側移動作用 (twosided translation)、共軛作用 (conjugation) あるいは随伴作用 (adjoint action) などと呼ばれ、この右作用版はよく冪記法を使って x^g= g⁻¹xg のように書かれる。これは (x^g)^h = x^ghを満足する。
  
  ・対称群 S_nとその部分群は、集合{ 1, ..., n } に元の置換として作用する。
  
  ・多面体の対称性の群は、多面体の頂点集合に作用し、多面体の面集合にも作用する。
  
  ・任意の幾何学的対象の対称性の群は、その対象の点集合の上に作用する。
  
  ・ベクトル空間、グラフ、群、環などの自己同型群はそれぞれ、そのベクトル空間、グラフの頂点集合、その群、その環などに作用する。
  
  ・一般線型群 GL(n, R), 特殊線型群 SL(n, R), 直交群 O(n,R) および特殊直交群 SO(n, R) は Rⁿに作用するリー群である。
  
  ・体の拡大 E/F のガロア群 Gal(E/F) は大きいほうの体 Eに作用する。ガロア群の任意の部分群も同様である。
  
  ・実数全体の成す加法群 (R, +) は古典力学︵およびもっと一般の力学系︶における﹁よく振舞う﹂系の相空間に作用する。これは Rの元 tと相空間の元 xに対して、系の状態を記述する xに対して、t • xは t秒後︵t が負なら t秒前︶の状態を表すものと定義することで得られる。
  
  ・実数全体の成す加法群 (R, +) は実函数全体の成す集合に作用する。作用 g• fはその任意の xにおける値を、たとえば
  ${\displaystyle f(x+g),\quad f($x)+g,\quad f(xe^{g}),\quad f(x)e^{g},\quad f(x+g)e^{g},\quad f(xe^{g})+g}  
  などと定めればよい。ただし f(xe^g + g) では作用にならない。
  
  ・絶対値が1の四元数全体は乗法群として R³に作用する。そのような任意の四元数
  $z=\cos(\alpha /2)+\sin(\alpha /2){\hat {\mathbf {v} }}$ 
  
  に対して、写像 f(x) = zxz^∗ は v-軸に関して反時計回りに角 α の回転を与える︵−z も同じ回転を与える︶。
  
  ・平面上の等長変換全体は平面画像や平面パターン全体の成す集合に作用する。これは、画像やパターンというものを︵例えば、色の集合に値をとる位置の函数であるといったように︶特定すればもう少し精密に定義ができる。
  
  ・より一般に、全単射 g: V→ Vからなる群は、写像 x: V→ W全体からなる集合に (gx)(v) = x(g⁻¹(v)) によって作用する︵全体でなくこの群作用について閉じているような写像の集合に制限して考えてもいい︶。従って、ある空間の全単射からなる群は、その空間に属する﹁物体﹂の集合への作用を誘導する。
  

  作用の種類

  
  群 Gの Xへの作用が、 
  
  ●推移的あるいは可移 (transitive) であるとは、X が空でなく、X の任意の元 xに対して Gx= Xが成り立つときに言う。ここで Gx= {gx | g∈ G} は xの Gによる軌道である。
  ●鋭推移的 (sharply transitive) であるとは Xの各元 yに対して、gx = yとなるような gが一意であるときにいう。これは後述の正則性と同値。
  
  ●n-重推移的 (n-transitive) であるとは、X が少なくとも n個の元を持ち、どの二つも相異なる任意の x₁, ..., x_nとどの二つも相異なる y₁, ..., y_nに対して g∈ Gで gx_k= y_k(1 ≤ k≤ n) が成り立つものが取れるときに言う。
  ●鋭 n-重推移的 (sharply n-transitive) であるとは、n-重推移的かつその定義における gがちょうど一つであるときにいう。
  
  ●忠実 (faithful) あるいは効果的 (effective) であるとは、G の相異なるどのような二元 g, hに対しても x∈ Xを適当に選べば gx≠ hxとなるようにできるときにいう。これは g≠ eなる Gの各元に対して x∈ Xで gx≠ xとなるものが存在するといっても同じことである。これは直観的には、G の異なる元が Xの異なる置換を引き起こすということを言っている。
  
  ●自由 (free) あるいは半正則 (semiregular) であるとは、X の任意の元 xに対して﹁ gx= hxとなるのは g= hであるときに限る﹂が成立することをいう。これは Xの任意の元 xに対して﹁ gx= xならば gは単位元である﹂が成り立つと言い換えてもよい。
  
  ●正則 (regular) あるいは単純推移的 (simply transitive) であるとは、自由かつ推移的であるときにいう。すなわち、X の任意の二元 x, yに対し、g ∈ Gがちょうど一つ存在して gx= yとできるということである。このとき、X は Gの主等質空間あるいは G-トーサーと呼ばれる。
  
  ●局所自由 (locally free) であるとは、G が位相群で、G の単位元 eの適当な近傍 Uが存在して、作用の Uへの制限が自由、すなわち Xの適当な元 xと Uの適当な元 gに対して　gx = xとなるならば g= eであることをいう。
  
  ●既約 (irreducible) であるとは、X がある環 R上の自明でない加群で、G の作用が R-線型であって、X は自明でない真の G-不変部分加群をもたないときにいう。
  
  空でない集合上の任意の自由作用は忠実である。群 Gの Xへの作用が忠実であるための必要十分条件は、群準同型 G→ Sym(X) の核が自明であることである。従って、G の Xへの忠実な作用があれば、G は X上の置換群のある部分群︵G の Sym(X) における像︶に同型である。
  
  任意の群 Gの左からの乗法による自身への作用は正則であり、したがって忠実でもある。従って、任意の群 Gはそれ自身の元上の対称群 Sym(G) に埋め込める︵これはケイリーの定理として知られる︶。
  
  群 Gが Xに忠実に作用しない場合も、群を少し変更して忠実作用を得ることができる。N = {g ∈ G| gx= x(∀x ∈ X)} と置けば、N は Gの正規部分群である︵実際、これは群準同型 G→ Sym(X) の核になっている︶。剰余群 G/N は (gN) • x := gxと置くことにより Xに忠実に作用する。X への Gのもともとの作用が忠実であることと N= {e} であることとは同値である。
  

  軌道と等方部分群

  

  In the compound of five tetrahedra, the symmetry group is the (rotational) icosahedral group I of order 60, while the stabilizer of a single chosen tetrahedron is the (rotational) tetrahedral group T of order 12, and the orbit space I/T (of order 60/12 = 5) is naturally identified with the 5 tetrahedra – the coset gT corresponds to which tetrahedron g sends the chosen tetrahedron to.

  
  群 Gが集合 Xに作用しているとき、X の点 xの軌道 (orbit) とは、G の各元を xに作用させた要素の集合である。x の軌道を Gxで表せば、
  
  $Gx=\left\{gx\mid g\in G\right\}$ 
  
  と書くことができる。群の性質から、X における︵各点の︶G の作用に関する軌道全体の成す集合が Xの類別を与えることが保証される。この類別に対応する同値関係 ∼ は﹁x ∼ yとなる必要十分条件は gx= yとなる g∈ Gが存在すること﹂として得られる。軌道はこの同値関係に関する同値類であり、二つの元 x, yが同値であることは、それらが属する軌道が一致 (Gx = Gy) することとして述べることもできる。
  
  G の作用に関する Xの軌道全体の成す集合は X/G︵あるいは多少稀だが G⧵X︶で表され、G の作用による Xの商 (quotient) とも呼ばれる。幾何学的な設定では軌道空間 (orbit space) とも、代数的な設定では余不変式 (coinvariant) の空間とも呼ばれ、X_G で表される︵これに対して不変式︵不動点︶の全体は X^Gで表される。余不変式の全体が﹁商﹂なのに対し、不変式の全体は﹁部分集合﹂となる︶。余不変式の概念と記法は特に群コホモロジーと群ホモロジー︵これも同様の添字の上付き・下付きで区別する慣習がある︶で用いられる。
  
  X の部分集合 Yに対し、
  
  $GY:=\{gy\mid y\in Y,\,g\in G\}$ 
  
  とする。部分集合 Yが Gの作用に関して安定あるいは不変 (invariant) であるとは、GY = Y︵これは GY⊆ Yとしても同じ︶が成り立つことを言う。このとき、G は Yにも作用している。また、部分集合 Yが Gの作用で固定される (fixed) あるいは Gが自明に作用するとは、G の各元 gと Yの各元 yに対して gy= yが成立することを言う。G の作用で固定される任意の部分群は G-不変だが、逆は正しくない。
  
  任意の軌道は、G が推移的に作用する Xの G-不変部分集合である。G の Xへの作用が推移的であるための必要十分条件は、全ての元が同値、すなわち軌道がただ一つであることである。
  
  X の各元 xに対して、x の安定化部分群あるいは固定部分群 (stabilizer subgroup)、等方部分群 (isotropy group) もしくは小群 (little group) などと呼ばれる Gの部分群を、x を固定する Gの元全体の成す集合
  
  $G_{x}=\{g\in G\mid gx=x\}$ 
  
  によって定める。これは Gの部分群だが、大抵は正規部分群でない。G の Xへの作用が自由であるための必要十分条件は、任意の固定部分群が自明であることである。群準同型 G→ Sym(X) の核 Nは、X の全ての元 xに関する固定部分群 G_xの交わりによって与えられる。
  
  軌道と固定部分群は近い関係にある。X の元 xを一つ固定して、写像
  
  $G\to X;\quad g\mapsto gx$ 
  
  を考える。この写像の像は xの属する軌道であり、余像は G_xの左剰余類全体の成す集合である。集合論における標準商定理により、 G/G_x と Gxとの間には自然な全単射が存在する。具体的にはこの全単射は hG_xと hxとの対応によって与えられる。このことは、軌道・固定部分群定理 (orbit-stabilizer theorem) として知られる。
  
  G と Xが共に有限ならば、軌道・固定部分群定理とラグランジュの定理から
  
  $|Gx|=[G:G_{x}]=|G|/|G_{x}|$ 
  
  が得られる。この結果はそれぞれの対象を数えることができるという点で特に有用である。
  
  二つの元 xおよび yが同じ軌道に属すならば、それらの固定部分群 G_xおよび G_yは互いに共軛であり、特に同型であることに注意。より詳しく、 G_gx= gG_xg⁻¹ が成立する。このように、互いに共軛な固定部分群を持つ点は、同じ軌道型 (orbit-type) を持つという。
  
  軌道・固定部分群定理に近い関係のある結果にバーンサイドの補題
  
  $\left|X/G\right|={\frac {1}{\left|G\right|}}\sum _{g\in G}\left|X^{g}\right|$ 
  
  がある。ここで X^gは gによって固定される Xの元全体の成す集合である。この結果は主に Gと Xが有限であるときに用いられ、軌道の総数は群の元ごとの不動点の数の平均に等しいことを示すものと解釈される。
  
  有限 G-集合の形式差 (formal difference) 全体の成す集合は、非交和を加法、直積を乗法として、バーンサイド環と呼ばれる環を成す。
  
  X の G-不変元 (invariant element) とは、G の全ての元に対して常に gx= xとなるような Xの元 xのことをいう。X の G-不変元の全体を X^Gで表して、X の G-不変部分集合と呼ぶ。X が G-加群であるときは、X^G は Gの Xに係数を持つ 0-次群コホモロジー群であり、高次のコホモロジー群は G-不変部分集合をとる函手の導来函手となる。
  

  群作用と亜群

  
  群作用の概念は、群作用に付随する﹁作用亜群﹂
  
  $G'=G\ltimes X$ 
  
  を対応させることによってより広い文脈において考えることができる。こうすることで、表示やファイバー付けといったような亜群の理論における手法が使えるようになる。さらに言えば、作用の固定化群は頂点群 (vertex group) であり、作用の軌道は作用亜群の成分である。詳細は (Ronald Brown 2006) を参照.
  
  この作用亜群には﹁亜群の被覆射﹂p: G′ → Gが考えられる。これにより、このような射と位相幾何学における被覆写像とが関連付けられる。
  

  射と同型

  
  X および Yがともに G-集合であるとき、X から Yへの G-集合の射あるいは準同型 (morphism) とは、写像 f: X→ Yであって、G の任意の元 gと Xの任意の元 xに対して
  
  ${\displaystyle f($gx)=gf(x)}  
  
  を満たすものを言う。G-集合の射は G-同変写像 (G-equivariant map) あるいは G-写像 (G-map) ともいう。
  
  そのような G-集合の射 fが全単射ならば、その逆写像も G-集合の射であり、f は G-集合の同型︵写像︶であるという。また、二つの G-集合 Xおよび Yは、その間に G-集合の同型写像が存在するとき、G-集合として同型 (isomorphic)であるといい、実用上は同じものとして区別されないことも多い。
  
  同型の例‥
  
  ●任意の正則 G-作用は Gの左からの乗法によって与えられる G自身への作用に同型である。
  
  ●任意の自由 G-作用は、ある集合 Sに対する G× Sに Gの作用を第一座標への左乗法によって定めたものに同型である。
  
  ●任意の推移的 G-作用は、G の適当な部分群 Hによる左剰余類全体の成す集合に Gの左からの乗法を考えたものに同型である。
  
  この射の概念を合わせて考えることにより、G-集合全体の集まりは圏を成す。この圏はグロタンディーク・トポスである︵実は古典メタ論理を仮定すれば、このトポスはブール的にもなる︶。
  

  連続な群作用

  
  G が位相群、X が位相空間であるとき、写像 G× X→ XがG × Xの積位相に関して連続であるような Gの Xへの連続群作用 (continuous group actions) を考えることもよくある。この場合、位相空間 Xを G-空間 (G-space) とも呼ぶ。任意の群は離散位相に関する位相群と見ることができるから、これは実際には一般化になっている。既に述べた各種概念はこの文脈でもそのまま考えることができるが、G-空間の間の射としては Gの作用と両立する﹁連続写像﹂を考えるのが普通である。商 X/G には Xから誘導される商位相を入れて位相空間としたものを、この作用に関する商空間 (quotient space) と呼ぶ。正則、自由、推移的な作用に対する同型射について上述した主張は、連続群作用に対してはもはや正しくない。 
  
  Gが位相空間 Xに作用する離散群であるとき、作用が固有不連続あるいは真性不連続 (properly discontinuous) であるのは、X の各点 xに対して開近傍 Uが存在して、g(U) ∩ U≠ ∅ となるような Gの元 g全体成す集合が、ただ一つ単位元のみからなるようにできるときである。X が別の位相空間 Yの正則被覆空間であるとき、デック変換群の Xへの作用は固有不連続かつ自由である。群 Gの弧状連結位相空間 Xへの、任意の自由かつ固有不連続な作用は、このようにして得られる。商写像 X↦ X/G は正則被覆写像であり、デック変換群は Gの Xへの作用によって与えられる。さらに、X が単連結ならば X/G の基本群は Gに同型である。これらの結果は (Brown 2006) で、適当な局所条件の下での、離散群のハウスドルフ空間への不連続作用の軌道空間の基本亜群や、空間の基本亜群の軌道亜群などを含む形に一般化されている。これにより、対称平方の基本群などが計算できるようになる。
  
  群 Gの局所コンパクト空間 Xへの作用が余コンパクト (cocompact) であるとは、X のコンパクト部分集合 Aで GA= Xとなるようなものが存在するときに言う。固有不連続作用に対しては、余コンパクト性は商空間 X/G のコンパクト性に同値である。
  
  G の Xへの作用が固有 (proper) であるとは、写像 G× X→ X× X; (g, x) ↦ (gx, x) is a 固有写像 (proper map) であるときに言う。
  

  強連続群作用と平滑点

  
  α: G× X→ Xを位相群 Gの位相空間 Xへの作用とする。作用 α が強連続 (strongly continuous) であるとは、X の各元 xに対して、写像 g↦ α_g(x) がそれぞれの位相に関して連続であるときに言う。このような作用は、X 上の連続写像全体の成す空間への Gの作用を
  
  ${\displaystyle (\alpha _{g}f)($x)=f(\alpha _{g}^{-1}x)}  
  
  によって誘導する。
  
  強連続作用 α に対する平滑点あるいはスムース点 (smooth points) とは、g ↦ α_g(x) が滑らか︵つまり、連続かつ各階の導函数が全て連続︶であるような Xの点 xのことをいう。
  

  一般化

  
  モノイドの集合への作用︵モノイド作用︶を、群作用と同じ二つの公理によって定義することができる。しかし、この場合は作用素が全単射となり同値関係を定めるというようなことは期待できない。
  
  集合への作用を考える代わりに、群やモノイドの適当な圏の対象への作用を考えることもできる。これはある圏の対象 Xからはじめて、X への作用を Xの自己準同型全体の成すモノイドへのモノイド準同型として定めたものである。対象 Xが台となる集合を持つならば、既に述べた各種の定義や結果はこの場合でも有効である。例えば、ベクトル空間の圏を考えることにより、この方法で群の表現が得られる。
  
  群 Gをすべての射が可逆な単一対象圏とみなせば、群作用とは Gから集合の圏 Set への函手、群の表現はベクトル空間の圏への函手に他ならない。同様に、G-集合の間の射は群作用函手の間の自然変換である。このアナロジーとして、亜群の作用を亜群から集合の圏あるいはもっと別の圏への函手として定義することができる。
  
  圏の言葉を使わずとも、集合 Xへの群の作用を、それが誘導する Xの冪集合2^X への作用を調べることによって拡張することもできる。これは例えば、24元集合上の巨大なマシュー群の作用や、有限幾何学のある種の模型の対称性を調べることなどに対して有用である。
  

  関連項目

  • 作用
  • 作用を持つ群
  • モノイド作用
  • Gain graph

  参考文献

  • Aschbacher, Michael (2000). Finite Group Theory (Second ed.). Cambridge University Press. ISBN 978-0-521-78675-1. MR1777008 
  • Brown, Ronald (2006). Topology and groupoids, Booksurge PLC, ISBN 1-4196-2722-8.
  • Categories and groupoids, P.J. Higgins, downloadable reprint of van Nostrand Notes in Mathematics, 1971, which deal with applications of groupoids in group theory and topology.
  • Dummit, David; Foote, Richard (2003). Abstract Algebra (3rd ed.). Wiley. ISBN 0-471-43334-9 
  • Rotman, Joseph (1995). An Introduction to the Theory of Groups. Graduate Texts in Mathematics 148 ((4th ed.) ed.). Springer-Verlag. ISBN 0-387-94285-8 

  • Weisstein, Eric W. "Group Action". mathworld.wolfram.com (英語).